Conjunto dominante de un gráfico

En teoría de grafos, un conjunto dominante para un gráfico G = (V, E) es un subconjunto D de V tal que cada vértice que no está en D es adyacente a al menos un miembro de D. El número de dominación es el número de vértices en un conjunto dominante más pequeño para G.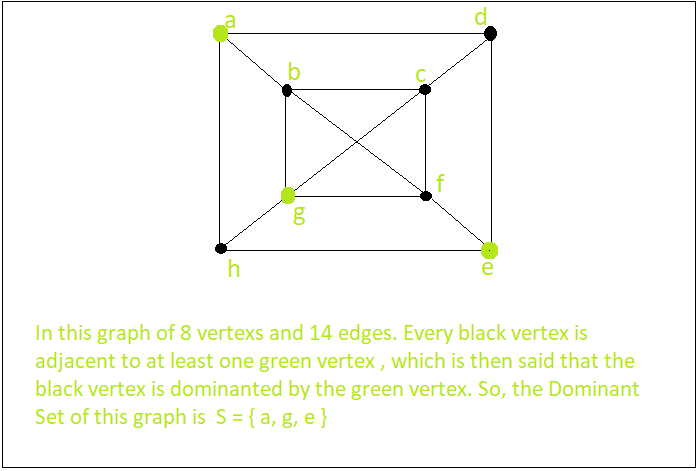

Ejemplos:

Input :   A graph with 4 vertex and 4 edges   
Output :  The Dominant Set S= { a, b } or { a, d } or { a, c } and more.

Input : A graph with 6 vertex and 7 edges   
Output :  The Dominant Set S= { a, d, f } or { e, c } and more.

Se cree que puede no haber un algoritmo eficiente que encuentre un conjunto dominante más pequeño para todos los gráficos, pero existen algoritmos de aproximación eficientes.
Algoritmo:

• Primero tenemos que inicializar un conjunto ‘S’ como vacío
• Tome cualquier borde ‘e’ del gráfico que conecta los vértices (digamos A y B)
• Agregue un vértice entre A y B (digamos A) a nuestro conjunto S
• Eliminar todos los bordes en el gráfico conectado a A
• Regrese al paso 2 y repita, si aún queda algún borde en el gráfico
• El conjunto final S es un Conjunto Dominante del grafo

// C++ program to find the Dominant Set of a graph
#include <bits/stdc++.h>
using namespace std;
  
vector<vector<int> > g;
bool box[100000];
  
vector<int> Dominant(int ver, int edge)
{
    vector<int> S; // set S
    for (int i = 0; i < ver; i++) {
        if (!box[i]) {
            S.push_back(i);
            box[i] = true;
            for (int j = 0; j < (int)g[i].size(); j++) {
                if (!box[g[i][j]]) {
                    box[g[i][j]] = true;
                    break;
                }
            }
        }
    }
    return S;
}
  
// Driver function
int main()
{
    int ver, edge, x, y;
  
    ver = 5; // Enter number of vertices
    edge = 6; // Enter number of Edges
    g.resize(ver);
  
    // Setting all index value of an array as 0
    memset(box, 0, sizeof(box)); 
  
    // Enter all the end-points of all the Edges
    // g[x--].push_back[y--]      g[y--].push_back[x--]
    g[0].push_back(1);
    g[1].push_back(0); // x = 1, y = 2 ;
    g[1].push_back(2);
    g[2].push_back(1); // x = 2, y = 3 ;
    g[2].push_back(3);
    g[3].push_back(2); // x = 3, y = 4 ;
    g[0].push_back(3);
    g[3].push_back(0); // x = 1, y = 4 ;
    g[3].push_back(4);
    g[4].push_back(3); // x = 4, y = 5 ;
    g[2].push_back(4);
    g[4].push_back(2); // x = 3, y = 5 ;
  
    vector<int> S = Dominant(ver, edge);
    cout << "The Dominant Set is : { ";
    for (int i = 0; i < (int)S.size(); i++)
        cout << S[i] + 1 << " ";
    cout << "}";
    return 0;
}

// Java program to find the Dominant Set of a graph
import java.util.*;
  
class GFG
{
  
static Vector<Integer> []g;
static boolean []box = new boolean[100000];
  
static Vector<Integer> Dominant(int ver, int edge)
{
    Vector<Integer> S = new Vector<Integer>(); // set S
    for (int i = 0; i < ver; i++) 
    {
        if (!box[i]) 
        {
            S.add(i);
            box[i] = true;
            for (int j = 0; j < (int)g[i].size(); j++) 
            {
                if (!box[g[i].get(j)])
                {
                    box[g[i].get(j)] = true;
                    break;
                }
            }
        }
    }
    return S;
}
  
// Driver code
public static void main(String[] args)
{
    int ver, edge, x, y;
  
    ver = 5; // Enter number of vertices
    edge = 6; // Enter number of Edges
    g = new Vector[ver];
    for (int i = 0; i < ver; i++)
        g[i] = new Vector<Integer>();
  
  
    // Enter all the end-points of all the Edges
    // g[x--].push_back[y--]     g[y--].push_back[x--]
    g[0].add(1);
    g[1].add(0); // x = 1, y = 2 ;
    g[1].add(2);
    g[2].add(1); // x = 2, y = 3 ;
    g[2].add(3);
    g[3].add(2); // x = 3, y = 4 ;
    g[0].add(3);
    g[3].add(0); // x = 1, y = 4 ;
    g[3].add(4);
    g[4].add(3); // x = 4, y = 5 ;
    g[2].add(4);
    g[4].add(2); // x = 3, y = 5 ;
  
    Vector<Integer> S = Dominant(ver, edge);
    System.out.print("The Dominant Set is : { ");
    for (int i = 0; i < (int)S.size(); i++)
        System.out.print(S.get(i) + 1 + " ");
    System.out.print("}");
}
}
  
// This code is contributed by Rajput-Ji

// C# program to find the Dominant Set of a graph
using System;
using System.Collections.Generic;
  
class GFG
{
  
static List<int> []g;
static bool []box = new bool[100000];
  
static List<int> Dominant(int ver, int edge)
{
    List<int> S = new List<int>(); // set S
    for (int i = 0; i < ver; i++) 
    {
        if (!box[i]) 
        {
            S.Add(i);
            box[i] = true;
            for (int j = 0; j < (int)g[i].Count; j++) 
            {
                if (!box[g[i][j]])
                {
                    box[g[i][j]] = true;
                    break;
                }
            }
        }
    }
    return S;
}
  
// Driver code
public static void Main(String[] args)
{
    int ver, edge;
  
    ver = 5; // Enter number of vertices
    edge = 6; // Enter number of Edges
    g = new List<int>[ver];
    for (int i = 0; i < ver; i++)
        g[i] = new List<int>();
  
    // Enter all the end-points of all the Edges
    // g[x--].push_back[y--]     g[y--].push_back[x--]
    g[0].Add(1);
    g[1].Add(0); // x = 1, y = 2 ;
    g[1].Add(2);
    g[2].Add(1); // x = 2, y = 3 ;
    g[2].Add(3);
    g[3].Add(2); // x = 3, y = 4 ;
    g[0].Add(3);
    g[3].Add(0); // x = 1, y = 4 ;
    g[3].Add(4);
    g[4].Add(3); // x = 4, y = 5 ;
    g[2].Add(4);
    g[4].Add(2); // x = 3, y = 5 ;
  
    List<int> S = Dominant(ver, edge);
    Console.Write("The Dominant Set is : { ");
    for (int i = 0; i < (int)S.Count; i++)
        Console.Write(S[i] + 1 + " ");
    Console.Write("}");
}
}
  
// This code is contributed by PrinciRaj1992

The Dominant Set is : { 1 3 5 }

Referencia: wiki