Requisito previo: conceptos básicos de LCA , unión de conjuntos disjuntos por rango y compresión de ruta
. Se nos da un árbol (se puede extender a un DAG) y tenemos muchas consultas de forma LCA (u, v), es decir, encontrar LCA de Nodes ‘u’ y ‘v’.
Podemos realizar esas consultas en tiempo O(N + QlogN) usando RMQ , donde O(N) tiempo para preprocesamiento y O(log N) para responder las consultas, donde
N = número de Nodes y
Q = número de consultas a ser respondido
¿Podemos hacerlo mejor que esto? ¿Podemos hacerlo en (casi) tiempo lineal? Sí.
El artículo presenta un algoritmo fuera de línea que realiza esas consultas en aproximadamente O (N + Q) tiempo. Aunque esto no es exactamente lineal, ya que hay una función inversa de Ackermann involucrada en el análisis de la complejidad del tiempo. Para obtener más detalles sobre la función inversa de Ackermann, consulte esto . Solo como resumen, podemos decir que la Función Inversa de Ackermann permanece menor que 4, para cualquier valor de tamaño de entrada que se pueda escribir en inversa física. Por lo tanto, consideramos esto como casi lineal.
Consideramos el árbol de entrada como se muestra a continuación. Procesaremos previamente el árbol y llenaremos dos arrays: child [] y sibling[] de acuerdo con la siguiente explicación :

Vamos a procesar estas consultas: LCA (5,4), LCA (1,3), LCA (2,3)
Ahora, después del preprocesamiento, realizamos una caminata LCA comenzando desde la raíz del árbol (aquí- Node ‘1’). Pero antes de la caminata LCA, coloreamos todos los Nodes con BLANCO . Durante toda la caminata LCA, usamos tres funciones de unión de conjuntos disjuntos: makeSet(), findSet(), unionSet().
Estas funciones utilizan la técnica de unión por rango y compresión de caminos para mejorar el tiempo de ejecución. Durante la caminata LCA, nuestras consultas se procesan y generan (en un orden aleatorio). Después de la caminata LCA de todo el árbol, todos los Nodes se colorean NEGRO .
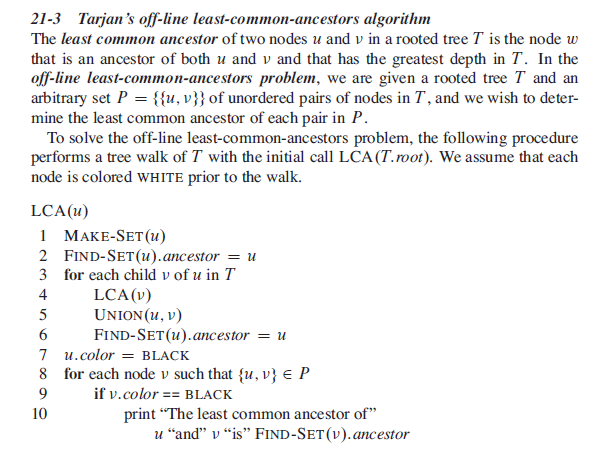
Tarjan Offline LCA Algorithm pasos de CLRS, Section-21-3, Pg 584, 2nd /3rd edition.
Nota- Es posible que las consultas no se procesen en el orden original. Podemos modificar fácilmente el proceso y ordenarlos según el orden de entrada.
Las siguientes imágenes muestran claramente todos los pasos que suceden. La flecha roja muestra la dirección de viaje de nuestra función recursiva LCA() .




Como podemos ver claramente en las imágenes de arriba, las consultas se procesan en el siguiente orden, LCA (5,4), LCA (2,3), LCA (1,3) que no está en el mismo orden que la entrada (LCA(5,4), LCA(1,3), LCA(2,3)).
A continuación se muestra la implementación del enfoque anterior:
C++
// A C++ Program to implement Tarjan Offline LCA Algorithm
#include <bits/stdc++.h>
#define V 5 // number of nodes in input tree
#define WHITE 1 // COLOUR 'WHITE' is assigned value 1
#define BLACK 2 // COLOUR 'BLACK' is assigned value 2
/* A binary tree node has data, pointer to left child
and a pointer to right child */
struct Node
{
int data;
Node* left, *right;
};
/*
subset[i].parent-->Holds the parent of node-'i'
subset[i].rank-->Holds the rank of node-'i'
subset[i].ancestor-->Holds the LCA queries answers
subset[i].child-->Holds one of the child of node-'i'
if present, else -'0'
subset[i].sibling-->Holds the right-sibling of node-'i'
if present, else -'0'
subset[i].color-->Holds the colour of node-'i'
*/
struct subset
{
int parent, rank, ancestor, child, sibling, color;
};
// Structure to represent a query
// A query consists of (L,R) and we will process the
// queries offline a/c to Tarjan's offline LCA algorithm
struct Query
{
int L, R;
};
/* Helper function that allocates a new node with the
given data and NULL left and right pointers. */
Node* newNode(int data)
{
Node* node = new Node;
node->data = data;
node->left = node->right = NULL;
return(node);
}
//A utility function to make set
void makeSet(struct subset subsets[], int i)
{
if (i < 1 || i > V)
return;
subsets[i].color = WHITE;
subsets[i].parent = i;
subsets[i].rank = 0;
return;
}
// A utility function to find set of an element i
// (uses path compression technique)
int findSet(struct subset subsets[], int i)
{
// find root and make root as parent of i (path compression)
if (subsets[i].parent != i)
subsets[i].parent = findSet (subsets, subsets[i].parent);
return subsets[i].parent;
}
// A function that does union of two sets of x and y
// (uses union by rank)
void unionSet(struct subset subsets[], int x, int y)
{
int xroot = findSet (subsets, x);
int yroot = findSet (subsets, y);
// Attach smaller rank tree under root of high rank tree
// (Union by Rank)
if (subsets[xroot].rank < subsets[yroot].rank)
subsets[xroot].parent = yroot;
else if (subsets[xroot].rank > subsets[yroot].rank)
subsets[yroot].parent = xroot;
// If ranks are same, then make one as root and increment
// its rank by one
else
{
subsets[yroot].parent = xroot;
(subsets[xroot].rank)++;
}
}
// The main function that prints LCAs. u is root's data.
// m is size of q[]
void lcaWalk(int u, struct Query q[], int m,
struct subset subsets[])
{
// Make Sets
makeSet(subsets, u);
// Initially, each node's ancestor is the node
// itself.
subsets[findSet(subsets, u)].ancestor = u;
int child = subsets[u].child;
// This while loop doesn't run for more than 2 times
// as there can be at max. two children of a node
while (child != 0)
{
lcaWalk(child, q, m, subsets);
unionSet (subsets, u, child);
subsets[findSet(subsets, u)].ancestor = u;
child = subsets[child].sibling;
}
subsets[u].color = BLACK;
for (int i = 0; i < m; i++)
{
if (q[i].L == u)
{
if (subsets[q[i].R].color == BLACK)
{
printf("LCA(%d %d) -> %d\n",
q[i].L,
q[i].R,
subsets[findSet(subsets,q[i].R)].ancestor);
}
}
else if (q[i].R == u)
{
if (subsets[q[i].L].color == BLACK)
{
printf("LCA(%d %d) -> %d\n",
q[i].L,
q[i].R,
subsets[findSet(subsets,q[i].L)].ancestor);
}
}
}
return;
}
// This is basically an inorder traversal and
// we preprocess the arrays-> child[]
// and sibling[] in "struct subset" with
// the tree structure using this function.
void preprocess(Node * node, struct subset subsets[])
{
if (node == NULL)
return;
// Recur on left child
preprocess(node->left, subsets);
if (node->left != NULL&&node->right != NULL)
{
/* Note that the below two lines can also be this-
subsets[node->data].child = node->right->data;
subsets[node->right->data].sibling =
node->left->data;
This is because if both left and right children of
node-'i' are present then we can store any of them
in subsets[i].child and correspondingly its sibling*/
subsets[node->data].child = node->left->data;
subsets[node->left->data].sibling =
node->right->data;
}
else if ((node->left != NULL && node->right == NULL)
|| (node->left == NULL && node->right != NULL))
{
if(node->left != NULL && node->right == NULL)
subsets[node->data].child = node->left->data;
else
subsets[node->data].child = node->right->data;
}
//Recur on right child
preprocess (node->right, subsets);
}
// A function to initialise prior to pre-processing and
// LCA walk
void initialise(struct subset subsets[])
{
// Initialising the structure with 0's
memset(subsets, 0, (V+1) * sizeof(struct subset));
// We colour all nodes WHITE before LCA Walk.
for (int i=1; i<=V; i++)
subsets[i].color=WHITE;
return;
}
// Prints LCAs for given queries q[0..m-1] in a tree
// with given root
void printLCAs(Node *root, Query q[], int m)
{
// Allocate memory for V subsets and nodes
struct subset * subsets = new subset[V+1];
// Creates subsets and colors them WHITE
initialise(subsets);
// Preprocess the tree
preprocess(root, subsets);
// Perform a tree walk to process the LCA queries
// offline
lcaWalk(root->data , q, m, subsets);
}
// Driver program to test above functions
int main()
{
/*
We construct a binary tree :-
1
/ \
2 3
/ \
4 5 */
Node *root = newNode(1);
root->left = newNode(2);
root->right = newNode(3);
root->left->left = newNode(4);
root->left->right = newNode(5);
// LCA Queries to answer
Query q[] = {{5, 4}, {1, 3}, {2, 3}};
int m = sizeof(q)/sizeof(q[0]);
printLCAs(root, q, m);
return 0;
}
Java
// A Java Program to implement Tarjan Offline LCA Algorithm
import java.util.Arrays;
class GFG
{
static final int V = 5; // number of nodes in input tree
static final int WHITE = 1; // COLOUR 'WHITE' is assigned value 1
static final int BLACK = 2; // COLOUR 'BLACK' is assigned value 2
/* A binary tree node has data, pointer to left child
and a pointer to right child */
static class Node
{
int data;
Node left, right;
};
/*
subset[i].parent-.Holds the parent of node-'i'
subset[i].rank-.Holds the rank of node-'i'
subset[i].ancestor-.Holds the LCA queries answers
subset[i].child-.Holds one of the child of node-'i'
if present, else -'0'
subset[i].sibling-.Holds the right-sibling of node-'i'
if present, else -'0'
subset[i].color-.Holds the colour of node-'i'
*/
static class subset
{
int parent;
int rank;
int ancestor;
int child;
int sibling;
int color;
};
// Structure to represent a query
// A query consists of (L,R) and we will process the
// queries offline a/c to Tarjan's offline LCA algorithm
static class Query
{
int L, R;
Query(int L, int R)
{
this.L = L;
this.R = R;
}
};
/* Helper function that allocates a new node with the
given data and null left and right pointers. */
static Node newNode(int data)
{
Node node = new Node();
node.data = data;
node.left = node.right = null;
return(node);
}
// A utility function to make set
static void makeSet(subset subsets[], int i)
{
if (i < 1 || i > V)
return;
subsets[i].color = WHITE;
subsets[i].parent = i;
subsets[i].rank = 0;
return;
}
// A utility function to find set of an element i
// (uses path compression technique)
static int findSet(subset subsets[], int i)
{
// find root and make root as parent of i (path compression)
if (subsets[i].parent != i)
subsets[i].parent = findSet (subsets, subsets[i].parent);
return subsets[i].parent;
}
// A function that does union of two sets of x and y
// (uses union by rank)
static void unionSet(subset subsets[], int x, int y)
{
int xroot = findSet (subsets, x);
int yroot = findSet (subsets, y);
// Attach smaller rank tree under root of high rank tree
// (Union by Rank)
if (subsets[xroot].rank < subsets[yroot].rank)
subsets[xroot].parent = yroot;
else if (subsets[xroot].rank > subsets[yroot].rank)
subsets[yroot].parent = xroot;
// If ranks are same, then make one as root and increment
// its rank by one
else
{
subsets[yroot].parent = xroot;
(subsets[xroot].rank)++;
}
}
// The main function that prints LCAs. u is root's data.
// m is size of q[]
static void lcaWalk(int u, Query q[], int m,
subset subsets[])
{
// Make Sets
makeSet(subsets, u);
// Initially, each node's ancestor is the node
// itself.
subsets[findSet(subsets, u)].ancestor = u;
int child = subsets[u].child;
// This while loop doesn't run for more than 2 times
// as there can be at max. two children of a node
while (child != 0)
{
lcaWalk(child, q, m, subsets);
unionSet (subsets, u, child);
subsets[findSet(subsets, u)].ancestor = u;
child = subsets[child].sibling;
}
subsets[u].color = BLACK;
for (int i = 0; i < m; i++)
{
if (q[i].L == u)
{
if (subsets[q[i].R].color == BLACK)
{
System.out.printf("LCA(%d %d)->%d\n",
q[i].L,
q[i].R,
subsets[findSet(subsets,q[i].R)].ancestor);
}
}
else if (q[i].R == u)
{
if (subsets[q[i].L].color == BLACK)
{
System.out.printf("LCA(%d %d)->%d\n",
q[i].L,
q[i].R,
subsets[findSet(subsets,q[i].L)].ancestor);
}
}
}
return;
}
// This is basically an inorder traversal and
// we preprocess the arrays. child[]
// and sibling[] in "subset" with
// the tree structure using this function.
static void preprocess(Node node, subset subsets[])
{
if (node == null)
return;
// Recur on left child
preprocess(node.left, subsets);
if (node.left != null && node.right != null)
{
/* Note that the below two lines can also be this-
subsets[node.data].child = node.right.data;
subsets[node.right.data].sibling =
node.left.data;
This is because if both left and right children of
node-'i' are present then we can store any of them
in subsets[i].child and correspondingly its sibling*/
subsets[node.data].child = node.left.data;
subsets[node.left.data].sibling =
node.right.data;
}
else if ((node.left != null && node.right == null)
|| (node.left == null && node.right != null))
{
if(node.left != null && node.right == null)
subsets[node.data].child = node.left.data;
else
subsets[node.data].child = node.right.data;
}
// Recur on right child
preprocess (node.right, subsets);
}
// A function to initialise prior to pre-processing and
// LCA walk
static void initialise(subset subsets[])
{
// We colour all nodes WHITE before LCA Walk.
for (int i = 1; i < subsets.length; i++)
{
subsets[i] = new subset();
subsets[i].color = WHITE;
}
return;
}
// Prints LCAs for given queries q[0..m-1] in a tree
// with given root
static void printLCAs(Node root, Query q[], int m)
{
// Allocate memory for V subsets and nodes
subset []subsets = new subset[V + 1];
// Creates subsets and colors them WHITE
initialise(subsets);
// Preprocess the tree
preprocess(root, subsets);
// Perform a tree walk to process the LCA queries
// offline
lcaWalk(root.data , q, m, subsets);
}
// Driver code
public static void main(String[] args)
{
/*
We construct a binary tree :-
1
/ \
2 3
/ \
4 5 */
Node root = newNode(1);
root.left = newNode(2);
root.right = newNode(3);
root.left.left = newNode(4);
root.left.right = newNode(5);
// LCA Queries to answer
Query q[] = new Query[3];
q[0] = new Query(5, 4);
q[1] = new Query(1, 3);
q[2] = new Query(2, 3);
int m = q.length;
printLCAs(root, q, m);
}
}
// This code is contributed by gauravrajput1
Python3
# A Python3 program to implement Tarjan
# Offline LCA Algorithm
# Number of nodes in input tree
V = 5
# COLOUR 'WHITE' is assigned value 1
WHITE = 1
# COLOUR 'BLACK' is assigned value 2
BLACK = 2
# A binary tree node has data, pointer
# to left child and a pointer to right child
class Node:
def __init__(self):
self.data = 0
self.left = None
self.right = None
'''
subset[i].parent-.Holds the parent of node-'i'
subset[i].rank-.Holds the rank of node-'i'
subset[i].ancestor-.Holds the LCA queries answers
subset[i].child-.Holds one of the child of node-'i'
if present, else -'0'
subset[i].sibling-.Holds the right-sibling of node-'i'
if present, else -'0'
subset[i].color-.Holds the colour of node-'i'
'''
class subset:
def __init__(self):
self.parent = 0
self.rank = 0
self.ancestor = 0
self.child = 0
self.sibling = 0
self.color = 0
# Structure to represent a query
# A query consists of (L,R) and we
# will process the queries offline
# a/c to Tarjan's offline LCA algorithm
class Query:
def __init__(self, L, R):
self.L = L
self.R = R
# Helper function that allocates a new node
# with the given data and None left and
# right pointers.
def newNode(data):
node = Node()
node.data = data
node.left = node.right = None
return (node)
# A utility function to make set
def makeSet(subsets, i):
if (i < 1 or i > V):
return
subsets[i].color = WHITE
subsets[i].parent = i
subsets[i].rank = 0
return
# A utility function to find set of an element i
# (uses path compression technique)
def findSet(subsets, i):
# Find root and make root as parent
# of i (path compression)
if (subsets[i].parent != i):
subsets[i].parent = findSet(subsets,
subsets[i].parent)
return subsets[i].parent
# A function that does union of two sets
# of x and y (uses union by rank)
def unionSet(subsets, x, y):
xroot = findSet(subsets, x)
yroot = findSet(subsets, y)
# Attach smaller rank tree under root of
# high rank tree (Union by Rank)
if (subsets[xroot].rank < subsets[yroot].rank):
subsets[xroot].parent = yroot
else if (subsets[xroot].rank > subsets[yroot].rank):
subsets[yroot].parent = xroot
# If ranks are same, then make one as root
# and increment its rank by one
else:
subsets[yroot].parent = xroot
(subsets[xroot].rank) += 1
# The main function that prints LCAs. u is
# root's data. m is size of q[]
def lcaWalk(u, q, m, subsets):
# Make Sets
makeSet(subsets, u)
# Initially, each node's ancestor is the node
# itself.
subsets[findSet(subsets, u)].ancestor = u
child = subsets[u].child
# This while loop doesn't run for more than 2 times
# as there can be at max. two children of a node
while (child != 0):
lcaWalk(child, q, m, subsets)
unionSet(subsets, u, child)
subsets[findSet(subsets, u)].ancestor = u
child = subsets[child].sibling
subsets[u].color = BLACK
for i in range(m):
if (q[i].L == u):
if (subsets[q[i].R].color == BLACK):
print("LCA(%d %d) -> %d" % (q[i].L, q[i].R,
subsets[findSet(subsets, q[i].R)].ancestor))
else if (q[i].R == u):
if (subsets[q[i].L].color == BLACK):
print("LCA(%d %d) -> %d" % (q[i].L, q[i].R,
subsets[findSet(subsets, q[i].L)].ancestor))
return
# This is basically an inorder traversal and
# we preprocess the arrays. child[]
# and sibling[] in "struct subset" with
# the tree structure using this function.
def preprocess(node, subsets):
if (node == None):
return
# Recur on left child
preprocess(node.left, subsets)
if (node.left != None and node.right != None):
''' Note that the below two lines can also be this-
subsets[node.data].child = node.right.data;
subsets[node.right.data].sibling =
node.left.data;
This is because if both left and right children of
node-'i' are present then we can store any of them
in subsets[i].child and correspondingly its sibling'''
subsets[node.data].child = node.left.data
subsets[node.left.data].sibling = node.right.data
else if ((node.left != None and node.right == None)
or (node.left == None and node.right != None)):
if (node.left != None and node.right == None):
subsets[node.data].child = node.left.data
else:
subsets[node.data].child = node.right.data
# Recur on right child
preprocess(node.right, subsets)
# A function to initialise prior to pre-processing and
# LCA walk
def initialise(subsets):
# Initialising the structure with 0's
# memset(subsets, 0, (V+1) * sizeof(struct subset));
# We colour all nodes WHITE before LCA Walk.
for i in range(1, V + 1):
subsets[i].color = WHITE
return
# Prints LCAs for given queries q[0..m-1] in a tree
# with given root
def printLCAs(root, q, m):
# Allocate memory for V subsets and nodes
subsets = [subset() for _ in range(V + 1)]
# Creates subsets and colors them WHITE
initialise(subsets)
# Preprocess the tree
preprocess(root, subsets)
# Perform a tree walk to process the LCA queries
# offline
lcaWalk(root.data, q, m, subsets)
# Driver code
if __name__ == "__main__":
'''
We construct a binary tree :-
1
/ \
2 3
/ \
4 5 '''
root = newNode(1)
root.left = newNode(2)
root.right = newNode(3)
root.left.left = newNode(4)
root.left.right = newNode(5)
# LCA Queries to answer
q = [Query(5, 4), Query(1, 3), Query(2, 3)]
m = len(q)
printLCAs(root, q, m)
# This code is contributed by sanjeev2552
C#
// A C# Program to implement Tarjan Offline LCA Algorithm
using System;
public class GFG
{
static readonly int V = 5; // number of nodes in input tree
static readonly int WHITE = 1; // COLOUR 'WHITE' is assigned value 1
static readonly int BLACK = 2; // COLOUR 'BLACK' is assigned value 2
/* A binary tree node has data, pointer to left child
and a pointer to right child */
public
class Node
{
public
int data;
public
Node left, right;
};
/*
subset[i].parent-.Holds the parent of node-'i'
subset[i].rank-.Holds the rank of node-'i'
subset[i].ancestor-.Holds the LCA queries answers
subset[i].child-.Holds one of the child of node-'i'
if present, else -'0'
subset[i].sibling-.Holds the right-sibling of node-'i'
if present, else -'0'
subset[i].color-.Holds the colour of node-'i'
*/
public
class subset
{
public
int parent;
public
int rank;
public
int ancestor;
public
int child;
public
int sibling;
public
int color;
};
// Structure to represent a query
// A query consists of (L,R) and we will process the
// queries offline a/c to Tarjan's offline LCA algorithm
public
class Query
{
public
int L, R;
public
Query(int L, int R)
{
this.L = L;
this.R = R;
}
};
/* Helper function that allocates a new node with the
given data and null left and right pointers. */
static Node newNode(int data)
{
Node node = new Node();
node.data = data;
node.left = node.right = null;
return(node);
}
// A utility function to make set
static void makeSet(subset []subsets, int i)
{
if (i < 1 || i > V)
return;
subsets[i].color = WHITE;
subsets[i].parent = i;
subsets[i].rank = 0;
return;
}
// A utility function to find set of an element i
// (uses path compression technique)
static int findSet(subset []subsets, int i)
{
// find root and make root as parent of i (path compression)
if (subsets[i].parent != i)
subsets[i].parent = findSet (subsets, subsets[i].parent);
return subsets[i].parent;
}
// A function that does union of two sets of x and y
// (uses union by rank)
static void unionSet(subset []subsets, int x, int y)
{
int xroot = findSet (subsets, x);
int yroot = findSet (subsets, y);
// Attach smaller rank tree under root of high rank tree
// (Union by Rank)
if (subsets[xroot].rank < subsets[yroot].rank)
subsets[xroot].parent = yroot;
else if (subsets[xroot].rank > subsets[yroot].rank)
subsets[yroot].parent = xroot;
// If ranks are same, then make one as root and increment
// its rank by one
else
{
subsets[yroot].parent = xroot;
(subsets[xroot].rank)++;
}
}
// The main function that prints LCAs. u is root's data.
// m is size of q[]
static void lcaWalk(int u, Query []q, int m,
subset []subsets)
{
// Make Sets
makeSet(subsets, u);
// Initially, each node's ancestor is the node
// itself.
subsets[findSet(subsets, u)].ancestor = u;
int child = subsets[u].child;
// This while loop doesn't run for more than 2 times
// as there can be at max. two children of a node
while (child != 0)
{
lcaWalk(child, q, m, subsets);
unionSet (subsets, u, child);
subsets[findSet(subsets, u)].ancestor = u;
child = subsets[child].sibling;
}
subsets[u].color = BLACK;
for (int i = 0; i < m; i++)
{
if (q[i].L == u)
{
if (subsets[q[i].R].color == BLACK)
{
Console.WriteLine("LCA(" + q[i].L + " " + q[i].R+") -> " +
subsets[findSet(subsets, q[i].R)].ancestor);
}
}
else if (q[i].R == u)
{
if (subsets[q[i].L].color == BLACK)
{
Console.WriteLine("LCA(" + q[i].L + " " + q[i].R + ") -> " +
subsets[findSet(subsets, q[i].L)].ancestor);
}
}
}
return;
}
// This is basically an inorder traversal and
// we preprocess the arrays. child[]
// and sibling[] in "subset" with
// the tree structure using this function.
static void preprocess(Node node, subset []subsets)
{
if (node == null)
return;
// Recur on left child
preprocess(node.left, subsets);
if (node.left != null && node.right != null)
{
/* Note that the below two lines can also be this-
subsets[node.data].child = node.right.data;
subsets[node.right.data].sibling =
node.left.data;
This is because if both left and right children of
node-'i' are present then we can store any of them
in subsets[i].child and correspondingly its sibling*/
subsets[node.data].child = node.left.data;
subsets[node.left.data].sibling =
node.right.data;
}
else if ((node.left != null && node.right == null)
|| (node.left == null && node.right != null))
{
if(node.left != null && node.right == null)
subsets[node.data].child = node.left.data;
else
subsets[node.data].child = node.right.data;
}
// Recur on right child
preprocess (node.right, subsets);
}
// A function to initialise prior to pre-processing and
// LCA walk
static void initialise(subset []subsets)
{
// We colour all nodes WHITE before LCA Walk.
for (int i = 1; i < subsets.Length; i++)
{
subsets[i] = new subset();
subsets[i].color = WHITE;
}
return;
}
// Prints LCAs for given queries q[0..m-1] in a tree
// with given root
static void printLCAs(Node root, Query []q, int m)
{
// Allocate memory for V subsets and nodes
subset []subsets = new subset[V + 1];
// Creates subsets and colors them WHITE
initialise(subsets);
// Preprocess the tree
preprocess(root, subsets);
// Perform a tree walk to process the LCA queries
// offline
lcaWalk(root.data, q, m, subsets);
}
// Driver code
public static void Main(String[] args)
{
/*
We construct a binary tree :-
1
/ \
2 3
/ \
4 5 */
Node root = newNode(1);
root.left = newNode(2);
root.right = newNode(3);
root.left.left = newNode(4);
root.left.right = newNode(5);
// LCA Queries to answer
Query []q = new Query[3];
q[0] = new Query(5, 4);
q[1] = new Query(1, 3);
q[2] = new Query(2, 3);
int m = q.Length;
printLCAs(root, q, m);
}
}
// This code is contributed by Rajput-Ji
Javascript
<script>
// A Javascript Program to implement Tarjan Offline LCA Algorithm
let V = 5; // number of nodes in input tree
let WHITE = 1; // COLOUR 'WHITE' is assigned value 1
let BLACK = 2; // COLOUR 'BLACK' is assigned value 2
/* A binary tree node has data, pointer to left child
and a pointer to right child */
class Node
{
constructor(data)
{
this.data = data;
this.left = this.right = null;
}
}
/*
subset[i].parent-.Holds the parent of node-'i'
subset[i].rank-.Holds the rank of node-'i'
subset[i].ancestor-.Holds the LCA queries answers
subset[i].child-.Holds one of the child of node-'i'
if present, else -'0'
subset[i].sibling-.Holds the right-sibling of node-'i'
if present, else -'0'
subset[i].color-.Holds the colour of node-'i'
*/
class subset
{
constructor()
{
this.parent = 0;
this.rank = 0;
this.ancestor = 0;
this.child = 0;
this.sibling = 0;
this.color = 0;
}
}
// Structure to represent a query
// A query consists of (L,R) and we will process the
// queries offline a/c to Tarjan's offline LCA algorithm
class Query
{
constructor(L, R)
{
this.L = L;
this.R = R;
}
}
/* Helper function that allocates a new node with the
given data and null left and right pointers. */
function newNode(data)
{
let node = new Node();
node.data = data;
node.left = node.right = null;
return(node);
}
// A utility function to make set
function makeSet(subsets,i)
{
if (i < 1 || i > V)
return;
subsets[i].color = WHITE;
subsets[i].parent = i;
subsets[i].rank = 0;
return;
}
// A utility function to find set of an element i
// (uses path compression technique)
function findSet(subsets, i)
{
// find root and make root as parent of i (path compression)
if (subsets[i].parent != i)
subsets[i].parent = findSet (subsets, subsets[i].parent);
return subsets[i].parent;
}
// A function that does union of two sets of x and y
// (uses union by rank)
function unionSet(subsets, x, y)
{
let xroot = findSet (subsets, x);
let yroot = findSet (subsets, y);
// Attach smaller rank tree under root of high rank tree
// (Union by Rank)
if (subsets[xroot].rank < subsets[yroot].rank)
subsets[xroot].parent = yroot;
else if (subsets[xroot].rank > subsets[yroot].rank)
subsets[yroot].parent = xroot;
// If ranks are same, then make one as root and increment
// its rank by one
else
{
subsets[yroot].parent = xroot;
(subsets[xroot].rank)++;
}
}
// The main function that prints LCAs. u is root's data.
// m is size of q[]
function lcaWalk(u,q,m,subsets)
{
// Make Sets
makeSet(subsets, u);
// Initially, each node's ancestor is the node
// itself.
subsets[findSet(subsets, u)].ancestor = u;
let child = subsets[u].child;
// This while loop doesn't run for more than 2 times
// as there can be at max. two children of a node
while (child != 0)
{
lcaWalk(child, q, m, subsets);
unionSet (subsets, u, child);
subsets[findSet(subsets, u)].ancestor = u;
child = subsets[child].sibling;
}
subsets[u].color = BLACK;
for (let i = 0; i < m; i++)
{
if (q[i].L == u)
{
if (subsets[q[i].R].color == BLACK)
{
document.write("LCA("+ q[i].L+" " +q[i].R+") -> ",subsets[findSet(subsets,q[i].R)].ancestor+"<br>");
}
}
else if (q[i].R == u)
{
if (subsets[q[i].L].color == BLACK)
{
document.write("LCA("+ q[i].L+" " +q[i].R+") -> ",subsets[findSet(subsets,q[i].L)].ancestor+"<br>");
}
}
}
return;
}
// This is basically an inorder traversal and
// we preprocess the arrays. child[]
// and sibling[] in "subset" with
// the tree structure using this function.
function preprocess(node,subsets)
{
if (node == null)
return;
// Recur on left child
preprocess(node.left, subsets);
if (node.left != null && node.right != null)
{
/* Note that the below two lines can also be this-
subsets[node.data].child = node.right.data;
subsets[node.right.data].sibling =
node.left.data;
This is because if both left and right children of
node-'i' are present then we can store any of them
in subsets[i].child and correspondingly its sibling*/
subsets[node.data].child = node.left.data;
subsets[node.left.data].sibling =
node.right.data;
}
else if ((node.left != null && node.right == null)
|| (node.left == null && node.right != null))
{
if(node.left != null && node.right == null)
subsets[node.data].child = node.left.data;
else
subsets[node.data].child = node.right.data;
}
// Recur on right child
preprocess (node.right, subsets);
}
// A function to initialise prior to pre-processing and
// LCA walk
function initialise(subsets)
{
// We colour all nodes WHITE before LCA Walk.
for (let i = 1; i < subsets.length; i++)
{
subsets[i] = new subset();
subsets[i].color = WHITE;
}
return;
}
// Prints LCAs for given queries q[0..m-1] in a tree
// with given root
function printLCAs(root, q, m)
{
// Allocate memory for V subsets and nodes
let subsets = new Array(V + 1);
// Creates subsets and colors them WHITE
initialise(subsets);
// Preprocess the tree
preprocess(root, subsets);
// Perform a tree walk to process the LCA queries
// offline
lcaWalk(root.data , q, m, subsets);
}
// Driver code
/*
We construct a binary tree :-
1
/ \
2 3
/ \
4 5 */
let root = newNode(1);
root.left = newNode(2);
root.right = newNode(3);
root.left.left = newNode(4);
root.left.right = newNode(5);
// LCA Queries to answer
let q = new Array(3);
q[0] = new Query(5, 4);
q[1] = new Query(1, 3);
q[2] = new Query(2, 3);
let m = q.length;
printLCAs(root, q, m);
// This code is contributed by patel2127
</script>
Producción :
LCA(5 4) -> 2 LCA(2 3) -> 1 LCA(1 3) -> 1
Complejidad de tiempo: superlineal, es decir, apenas más lento que lineal. O(N + Q) tiempo, donde O(N) tiempo de preprocesamiento y casi O(1) tiempo de respuesta a las consultas.
Espacio auxiliar: usamos muchas arrays: padre [], rango [], antepasado [] que se usan en operaciones de unión de conjuntos disjuntos, cada uno con el tamaño igual al número de Nodes. También usamos las arrays: child[], sibling[], color[], que son útiles en este algoritmo fuera de línea. Por lo tanto, usamos O(N).
Por comodidad, todos estos arreglos se colocan en un subconjunto de estructura-estructura para contener estos arreglos.
Referencias
https://en.wikipedia.org/wiki/Tarjan%27s_off-line_lowest_common_ancestors_algorithm
CLRS, Section-21-3, Pg 584, 2nd /3rd edition
http://wcipeg.com/wiki/Lowest_common_ancestor#Offline
Este artículo es una contribución por Rachit Belwariar . Si le gusta GeeksforGeeks y le gustaría contribuir, también puede escribir un artículo y enviarlo por correo a review-team@geeksforgeeks.org. Vea su artículo que aparece en la página principal de GeeksforGeeks y ayude a otros Geeks.
Escriba comentarios si encuentra algo incorrecto o si desea compartir más información sobre el tema tratado anteriormente.
Publicación traducida automáticamente
Artículo escrito por GeeksforGeeks-1 y traducido por Barcelona Geeks. The original can be accessed here. Licence: CCBY-SA