Dado un texto txt[0..n-1] y un patrón pat[0..m-1] , escriba una función search(char pat[], char txt[]) que imprima todas las apariciones de pat[] en txt [] . Puede suponer que n > m .
Ejemplos:
Input: txt[] = "THIS IS A TEST TEXT"
pat[] = "TEST"
Output: Pattern found at index 10
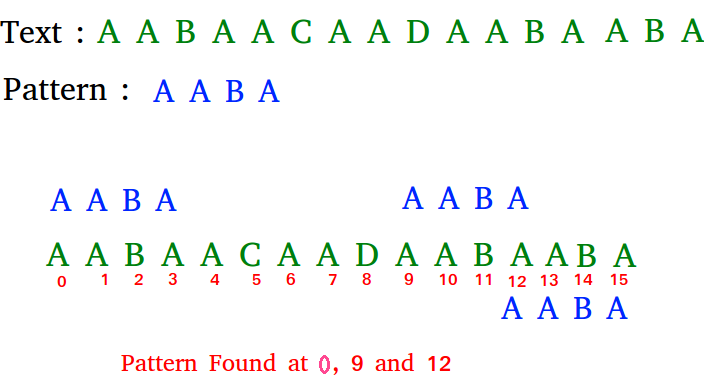
Input: txt[] = "AABAACAADAABAABA"
pat[] = "AABA"
Output: Pattern found at index 0
Pattern found at index 9
Pattern found at index 12
La búsqueda de patrones es un problema importante en informática. Cuando buscamos una string en el bloc de notas/archivo de Word, en el navegador o en la base de datos, se utilizan algoritmos de búsqueda de patrones para mostrar los resultados de la búsqueda.
Python3
# Python program for KMP Algorithm
def KMPSearch(pat, txt):
M = len(pat)
N = len(txt)
# create lps[] that will hold the longest prefix suffix
# values for pattern
lps = [0]*M
j = 0 # index for pat[]
# Preprocess the pattern (calculate lps[] array)
computeLPSArray(pat, M, lps)
i = 0 # index for txt[]
while i < N:
if pat[j] == txt[i]:
i += 1
j += 1
if j == M:
print ("Found pattern at index", str(i-j))
j = lps[j-1]
# mismatch after j matches
elif i < N and pat[j] != txt[i]:
# Do not match lps[0..lps[j-1]] characters,
# they will match anyway
if j != 0:
j = lps[j-1]
else:
i += 1
def computeLPSArray(pat, M, lps):
len = 0 # length of the previous longest prefix suffix
lps[0] # lps[0] is always 0
i = 1
# the loop calculates lps[i] for i = 1 to M-1
while i < M:
if pat[i]== pat[len]:
len += 1
lps[i] = len
i += 1
else:
# This is tricky. Consider the example.
# AAACAAAA and i = 7. The idea is similar
# to search step.
if len != 0:
len = lps[len-1]
# Also, note that we do not increment i here
else:
lps[i] = 0
i += 1
txt = "ABABDABACDABABCABAB"
pat = "ABABCABAB"
KMPSearch(pat, txt)
# This code is contributed by Bhavya Jain
Found pattern at index 10
Complejidad del tiempo : O(m+n)
Complejidad espacial : O(m)
Consulte el artículo completo sobre el algoritmo KMP para la búsqueda de patrones para obtener más detalles.
Publicación traducida automáticamente
Artículo escrito por GeeksforGeeks-1 y traducido por Barcelona Geeks. The original can be accessed here. Licence: CCBY-SA