Hemos visto diferentes formas de realizar el recorrido posterior al pedido en árboles binarios.
- Transversal de Post Orden .
- Recorrido iterativo en posorden utilizando dos pilas .
- Recorrido iterativo posterior al pedido utilizando One Stack .
Aquí hay otra forma de realizar el recorrido posorden en un árbol binario iterativamente usando una sola pila.
Considere las siguientes terminologías:
0 - Left element 1 - Right element 2 - Node element
El siguiente es el algoritmo detallado:
Take a Stack and perform the below operations:
1) Insert a pair of the root node as (node, 0).
2) Pop the top element to get the pair
(Let a = node and b be the variable)
If b is equal to 0:
Push another pair as (node, 1) and
Push the left child as (node->left, 0)
Repeat Step 2
Else If b is equal to 1:
Push another pair as (node, 2) and
Push right child of node as (node->right, 0)
Repeat Step 2
Else If b is equal to 2:
Print(node->data)
3) Repeat the above steps while stack is not empty
Considere el siguiente árbol binario con solo 3 Nodes:
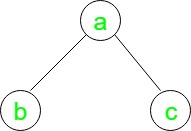
Ilustración:
1) Push(a, 0)
Stack - (a, 0)
2) top = (a, 0)
Push(a, 1)
Push(b, 0)
Stack - (b, 0)
(a, 1)
3) top = (b, 0)
Push(b, 1)
Stack - (b, 1)
(a, 1)
4) top = (b, 1)
Push(b, 2)
Stack - (b, 2)
(a, 1)
5) top = (b, 2)
print(b)
Stack -(a, 1)
6) top = (a, 1)
push(a, 2)
push(c, 0)
Stack - (c, 0)
(a, 2)
7) top = (c, 0)
push(c, 1)
Stack - (c, 1)
(a, 2)
8) top = (c, 1)
push(c, 2)
Stack - (c, 2)
(a, 2)
9) top = (c, 2)
print(c)
Stack - (a, 2)
10) top = (a, 2)
print(a)
Stack - empty()
A continuación se muestra la implementación del enfoque anterior:
C++
// C++ program to print postorder traversal
// iteratively
#include <bits/stdc++.h>
using namespace std;
// Binary Tree Node
struct Node {
int data;
struct Node* left;
struct Node* right;
};
// Helper function to create a
// new Binary Tree node
struct Node* newNode(int data)
{
struct Node* node = new Node;
node->data = data;
node->left = NULL;
node->right = NULL;
return (node);
}
// Function to perform postorder
// traversal iteratively
void iterativePostorder(Node* root)
{
stack<pair<Node*, int> > st;
st.push(make_pair(root, 0));
while (!st.empty()) {
struct Node* temp = st.top().first;
int b = st.top().second;
st.pop();
if (temp == NULL)
continue;
if (b == 0) {
st.push(make_pair(temp, 1));
if (temp->left != NULL)
st.push(make_pair(temp->left, 0));
}
else if (b == 1) {
st.push(make_pair(temp, 2));
if (temp->right != NULL)
st.push(make_pair(temp->right, 0));
}
else
cout << temp->data << " ";
}
}
// Driver Code
int main()
{
// Construct the below Binary Tree
// 1
// / \
// 2 3
// / \
// 4 5
Node* root = newNode(1);
root->left = newNode(2);
root->right = newNode(3);
root->left->left = newNode(4);
root->left->right = newNode(5);
iterativePostorder(root);
return 0;
}
Java
// Java program to print postorder traversal
// iteratively
import java.util.*;
class GFG
{
//pair class
static class Pair
{
Node first;
int second;
Pair(Node a,int b)
{
first = a;
second = b;
}
}
// Binary Tree Node
static class Node
{
int data;
Node left;
Node right;
};
// Helper function to create a
// new Binary Tree node
static Node newNode(int data)
{
Node node = new Node();
node.data = data;
node.left = null;
node.right = null;
return (node);
}
// Function to perform postorder
// traversal iteratively
static void iterativePostorder(Node root)
{
Stack<Pair> st = new Stack<Pair>();
st.add(new Pair(root, 0));
while (st.size() > 0)
{
Node temp = st.peek().first;
int b = st.peek().second;
st.pop();
if (temp == null)
continue;
if (b == 0)
{
st.add(new Pair(temp, 1));
if (temp.left != null)
st.add(new Pair(temp.left, 0));
}
else if (b == 1)
{
st.add(new Pair(temp, 2));
if (temp.right != null)
st.add(new Pair(temp.right, 0));
}
else
System.out.print( temp.data + " ");
}
}
// Driver Code
public static void main(String args[])
{
// Construct the below Binary Tree
// 1
// / \
// 2 3
// / \
// 4 5
Node root = newNode(1);
root.left = newNode(2);
root.right = newNode(3);
root.left.left = newNode(4);
root.left.right = newNode(5);
iterativePostorder(root);
}
}
// This code is contributed by Arnab Kundu
Python3
# Python program to print postorder traversal # iteratively # pair class class Pair: def __init__(self, a, b): self.first = a self.second = b # Binary Tree Node class Node : def __init__(self): self.data = 0 self.left = None self.right = None # Helper function to create a # new Binary Tree node def newNode(data): node = Node() node.data = data node.left = None node.right = None return (node) # Function to perform postorder # traversal iteratively def iterativePostorder( root): st = [] st.append(Pair(root, 0)) while (len(st)> 0): temp = st[-1].first b = st[-1].second st.pop() if (temp == None): continue if (b == 0) : st.append(Pair(temp, 1)) if (temp.left != None): st.append(Pair(temp.left, 0)) elif (b == 1): st.append(Pair(temp, 2)) if (temp.right != None): st.append(Pair(temp.right, 0)) else: print( temp.data ,end= " ") # Driver Code # Construct the below Binary Tree # 1 # / \ # 2 3 # / \ # 4 5 root = newNode(1) root.left = newNode(2) root.right = newNode(3) root.left.left = newNode(4) root.left.right = newNode(5) iterativePostorder(root) # This code is contributed by Arnab Kundu
C#
// C# program to print postorder traversal
// iteratively
using System;
using System.Collections.Generic;
class GFG
{
//pair class
public class Pair
{
public Node first;
public int second;
public Pair(Node a,int b)
{
first = a;
second = b;
}
}
// Binary Tree Node
public class Node
{
public int data;
public Node left;
public Node right;
};
// Helper function to create a
// new Binary Tree node
static Node newNode(int data)
{
Node node = new Node();
node.data = data;
node.left = null;
node.right = null;
return (node);
}
// Function to perform postorder
// traversal iteratively
static void iterativePostorder(Node root)
{
Stack<Pair> st = new Stack<Pair>();
st.Push(new Pair(root, 0));
while (st.Count > 0)
{
Node temp = st.Peek().first;
int b = st.Peek().second;
st.Pop();
if (temp == null)
continue;
if (b == 0)
{
st.Push(new Pair(temp, 1));
if (temp.left != null)
st.Push(new Pair(temp.left, 0));
}
else if (b == 1)
{
st.Push(new Pair(temp, 2));
if (temp.right != null)
st.Push(new Pair(temp.right, 0));
}
else
Console.Write(temp.data + " ");
}
}
// Driver Code
public static void Main(String []args)
{
// Construct the below Binary Tree
// 1
// / \
// 2 3
// / \
// 4 5
Node root = newNode(1);
root.left = newNode(2);
root.right = newNode(3);
root.left.left = newNode(4);
root.left.right = newNode(5);
iterativePostorder(root);
}
}
// This code is contributed by 29AjayKumar
Javascript
<script>
// JavaScript program to print
// postorder traversal iteratively
// Binary Tree Node
class Node
{
constructor(data) {
this.left = null;
this.right = null;
this.data = data;
}
}
// Helper function to create a
// new Binary Tree node
function newNode(data)
{
let node = new Node(data);
return (node);
}
// Function to perform postorder
// traversal iteratively
function iterativePostorder(root)
{
let st = [];
st.push([root, 0]);
while (st.length > 0)
{
let temp = st[st.length - 1][0];
let b = st[st.length - 1][1];
st.pop();
if (temp == null)
continue;
if (b == 0)
{
st.push([temp, 1]);
if (temp.left != null)
st.push([temp.left, 0]);
}
else if (b == 1)
{
st.push([temp, 2]);
if (temp.right != null)
st.push([temp.right, 0]);
}
else
document.write(temp.data + " ");
}
}
// Construct the below Binary Tree
// 1
// / \
// 2 3
// / \
// 4 5
let root = newNode(1);
root.left = newNode(2);
root.right = newNode(3);
root.left.left = newNode(4);
root.left.right = newNode(5);
iterativePostorder(root);
</script>
Producción:
4 5 2 3 1
Complejidad temporal: O(N)
Espacio auxiliar: O(N)
Publicación traducida automáticamente
Artículo escrito por everythingispossible y traducido por Barcelona Geeks. The original can be accessed here. Licence: CCBY-SA