La ordenación topológica para un gráfico cíclico dirigido ( DAG ) es una ordenación lineal de vértices tal que para cada arista dirigida uv, el vértice u viene antes que v en la ordenación. La clasificación topológica de un gráfico no es posible si el gráfico no es un DAG.
Dado un DAG, imprima todos los tipos topológicos del gráfico.
For example, consider the below graph.
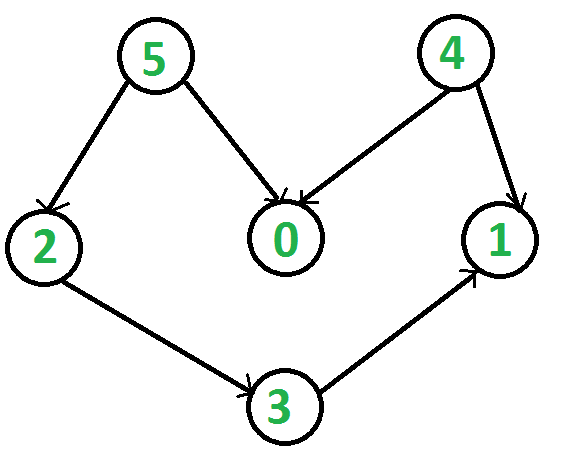
All topological sorts of the given graph are: 4 5 0 2 3 1 4 5 2 0 3 1 4 5 2 3 0 1 4 5 2 3 1 0 5 2 3 4 0 1 5 2 3 4 1 0 5 2 4 0 3 1 5 2 4 3 0 1 5 2 4 3 1 0 5 4 0 2 3 1 5 4 2 0 3 1 5 4 2 3 0 1 5 4 2 3 1 0
En un gráfico acíclico dirigido, muchas veces podemos tener vértices que no están relacionados entre sí, por lo que podemos ordenarlos de muchas maneras. Esta clasificación topológica diversa es importante en muchos casos, por ejemplo, si también hay algún peso relativo disponible entre los vértices, que es minimizar, entonces debemos cuidar el orden relativo y su peso relativo, lo que crea la necesidad de verificar todos los posibles ordenamientos topológicos.
Podemos pasar por todos los pedidos posibles a través de backtracking, el paso del algoritmo es el siguiente:
- Inicialice todos los vértices como no visitados.
- Ahora elija el vértice que no está visitado y tiene un grado de entrada cero y disminuya el grado de todos esos vértices en 1 (correspondiente a la eliminación de bordes) ahora agregue este vértice al resultado y llame a la función recursiva nuevamente y retroceda.
- Después de regresar de la función, restablezca los valores de visitado, resultado y grado de entrada para la enumeración de otras posibilidades.
A continuación se muestra la implementación de los pasos anteriores.
C++
// C++ program to print all topological sorts of a graph
#include <bits/stdc++.h>
using namespace std;
class Graph
{
int V; // No. of vertices
// Pointer to an array containing adjacency list
list<int> *adj;
// Vector to store indegree of vertices
vector<int> indegree;
// A function used by alltopologicalSort
void alltopologicalSortUtil(vector<int>& res,
bool visited[]);
public:
Graph(int V); // Constructor
// function to add an edge to graph
void addEdge(int v, int w);
// Prints all Topological Sorts
void alltopologicalSort();
};
// Constructor of graph
Graph::Graph(int V)
{
this->V = V;
adj = new list<int>[V];
// Initialising all indegree with 0
for (int i = 0; i < V; i++)
indegree.push_back(0);
}
// Utility function to add edge
void Graph::addEdge(int v, int w)
{
adj[v].push_back(w); // Add w to v's list.
// increasing inner degree of w by 1
indegree[w]++;
}
// Main recursive function to print all possible
// topological sorts
void Graph::alltopologicalSortUtil(vector<int>& res,
bool visited[])
{
// To indicate whether all topological are found
// or not
bool flag = false;
for (int i = 0; i < V; i++)
{
// If indegree is 0 and not yet visited then
// only choose that vertex
if (indegree[i] == 0 && !visited[i])
{
// reducing indegree of adjacent vertices
list<int>:: iterator j;
for (j = adj[i].begin(); j != adj[i].end(); j++)
indegree[*j]--;
// including in result
res.push_back(i);
visited[i] = true;
alltopologicalSortUtil(res, visited);
// resetting visited, res and indegree for
// backtracking
visited[i] = false;
res.erase(res.end() - 1);
for (j = adj[i].begin(); j != adj[i].end(); j++)
indegree[*j]++;
flag = true;
}
}
// We reach here if all vertices are visited.
// So we print the solution here
if (!flag)
{
for (int i = 0; i < res.size(); i++)
cout << res[i] << " ";
cout << endl;
}
}
// The function does all Topological Sort.
// It uses recursive alltopologicalSortUtil()
void Graph::alltopologicalSort()
{
// Mark all the vertices as not visited
bool *visited = new bool[V];
for (int i = 0; i < V; i++)
visited[i] = false;
vector<int> res;
alltopologicalSortUtil(res, visited);
}
// Driver program to test above functions
int main()
{
// Create a graph given in the above diagram
Graph g(6);
g.addEdge(5, 2);
g.addEdge(5, 0);
g.addEdge(4, 0);
g.addEdge(4, 1);
g.addEdge(2, 3);
g.addEdge(3, 1);
cout << "All Topological sorts\n";
g.alltopologicalSort();
return 0;
}
Java
//Java program to print all topological sorts of a graph
import java.util.*;
class Graph {
int V; // No. of vertices
List<Integer> adjListArray[];
public Graph(int V) {
this.V = V;
@SuppressWarnings("unchecked")
List<Integer> adjListArray[] = new LinkedList[V];
this.adjListArray = adjListArray;
for (int i = 0; i < V; i++) {
adjListArray[i] = new LinkedList<>();
}
}
// Utility function to add edge
public void addEdge(int src, int dest) {
this.adjListArray[src].add(dest);
}
// Main recursive function to print all possible
// topological sorts
private void allTopologicalSortsUtil(boolean[] visited,
int[] indegree, ArrayList<Integer> stack) {
// To indicate whether all topological are found
// or not
boolean flag = false;
for (int i = 0; i < this.V; i++) {
// If indegree is 0 and not yet visited then
// only choose that vertex
if (!visited[i] && indegree[i] == 0) {
// including in result
visited[i] = true;
stack.add(i);
for (int adjacent : this.adjListArray[i]) {
indegree[adjacent]--;
}
allTopologicalSortsUtil(visited, indegree, stack);
// resetting visited, res and indegree for
// backtracking
visited[i] = false;
stack.remove(stack.size() - 1);
for (int adjacent : this.adjListArray[i]) {
indegree[adjacent]++;
}
flag = true;
}
}
// We reach here if all vertices are visited.
// So we print the solution here
if (!flag) {
stack.forEach(i -> System.out.print(i + " "));
System.out.println();
}
}
// The function does all Topological Sort.
// It uses recursive alltopologicalSortUtil()
public void allTopologicalSorts() {
// Mark all the vertices as not visited
boolean[] visited = new boolean[this.V];
int[] indegree = new int[this.V];
for (int i = 0; i < this.V; i++) {
for (int var : this.adjListArray[i]) {
indegree[var]++;
}
}
ArrayList<Integer> stack = new ArrayList<>();
allTopologicalSortsUtil(visited, indegree, stack);
}
// Driver code
public static void main(String[] args) {
// Create a graph given in the above diagram
Graph graph = new Graph(6);
graph.addEdge(5, 2);
graph.addEdge(5, 0);
graph.addEdge(4, 0);
graph.addEdge(4, 1);
graph.addEdge(2, 3);
graph.addEdge(3, 1);
System.out.println("All Topological sorts");
graph.allTopologicalSorts();
}
}
Python3
# class to represent a graph object
class Graph:
# Constructor
def __init__(self, edges, N):
# A List of Lists to represent an adjacency list
self.adjList = [[] for _ in range(N)]
# stores in-degree of a vertex
# initialize in-degree of each vertex by 0
self.indegree = [0] * N
# add edges to the undirected graph
for (src, dest) in edges:
# add an edge from source to destination
self.adjList[src].append(dest)
# increment in-degree of destination vertex by 1
self.indegree[dest] = self.indegree[dest] + 1
# Recursive function to find
# all topological orderings of a given DAG
def findAllTopologicalOrders(graph, path, discovered, N):
# do for every vertex
for v in range(N):
# proceed only if in-degree of current node is 0 and
# current node is not processed yet
if graph.indegree[v] == 0 and not discovered[v]:
# for every adjacent vertex u of v,
# reduce in-degree of u by 1
for u in graph.adjList[v]:
graph.indegree[u] = graph.indegree[u] - 1
# include current node in the path
# and mark it as discovered
path.append(v)
discovered[v] = True
# recur
findAllTopologicalOrders(graph, path, discovered, N)
# backtrack: reset in-degree
# information for the current node
for u in graph.adjList[v]:
graph.indegree[u] = graph.indegree[u] + 1
# backtrack: remove current node from the path and
# mark it as undiscovered
path.pop()
discovered[v] = False
# print the topological order if
# all vertices are included in the path
if len(path) == N:
print(path)
# Print all topological orderings of a given DAG
def printAllTopologicalOrders(graph):
# get number of nodes in the graph
N = len(graph.adjList)
# create an auxiliary space to keep track of whether vertex is discovered
discovered = [False] * N
# list to store the topological order
path = []
# find all topological ordering and print them
findAllTopologicalOrders(graph, path, discovered, N)
# Driver code
if __name__ == '__main__':
# List of graph edges as per above diagram
edges = [(5, 2), (5, 0), (4, 0), (4, 1), (2, 3), (3, 1)]
print("All Topological sorts")
# Number of nodes in the graph
N = 6
# create a graph from edges
graph = Graph(edges, N)
# print all topological ordering of the graph
printAllTopologicalOrders(graph)
# This code is contributed by Priyadarshini Kumari
All Topological sorts 4 5 0 2 3 1 4 5 2 0 3 1 4 5 2 3 0 1 4 5 2 3 1 0 5 2 3 4 0 1 5 2 3 4 1 0 5 2 4 0 3 1 5 2 4 3 0 1 5 2 4 3 1 0 5 4 0 2 3 1 5 4 2 0 3 1 5 4 2 3 0 1 5 4 2 3 1 0
Este artículo es una contribución de Utkarsh Trivedi. Escriba comentarios si encuentra algo incorrecto o si desea compartir más información sobre el tema tratado anteriormente.
Publicación traducida automáticamente
Artículo escrito por GeeksforGeeks-1 y traducido por Barcelona Geeks. The original can be accessed here. Licence: CCBY-SA