Dado el valor de sin(Θ) y una variable n<=15. La tarea es encontrar el valor de sin(nΘ) usando la propiedad de las funciones trigonométricas.
Ejemplos :
Input: sin(Θ)=0.5, n=1 Output: 0.5 Input: sin(Θ)=0.5, n=10 Output: -0.866025
Enfoque: Este problema se puede resolver usando el teorema de De moivre y el teorema del binomio
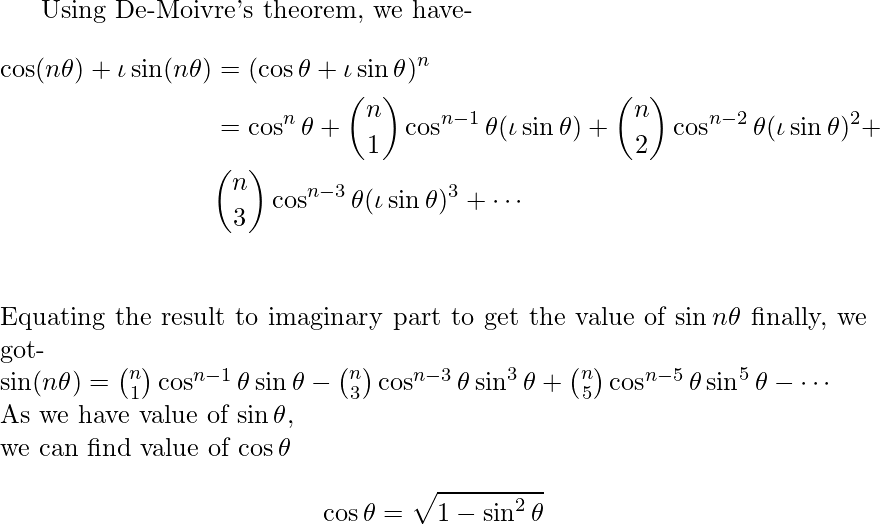
Ahora, tenemos tanto sen(Θ) como cos(Θ). Pon el valor en la ecuación para obtener tu respuesta.
A continuación se muestra la implementación del enfoque anterior:
C++
// C++ Program to find the value of sin(n?)
#include <bits/stdc++.h>
#define ll long long int
#define MAX 16
using namespace std;
ll nCr[MAX][MAX] = { 0 };
// This function use to calculate the
// binomial coefficient upto 15
void binomial()
{
// use simple DP to find coefficient
for (int i = 0; i < MAX; i++) {
for (int j = 0; j <= i; j++) {
if (j == 0 || j == i)
nCr[i][j] = 1;
else
nCr[i][j] = nCr[i - 1][j] + nCr[i - 1][j - 1];
}
}
}
// Function to find the value of
double findCosNTheta(double sinTheta, ll n)
{
// find cosTheta from sinTheta
double cosTheta = sqrt(1 - sinTheta * sinTheta);
// store required answer
double ans = 0;
// use to toggle sign in sequence.
ll toggle = 1;
for (int i = 1; i <= n; i += 2) {
ans = ans + nCr[n][i] * pow(cosTheta, n - i)
* pow(sinTheta, i) * toggle;
toggle = toggle * -1;
}
return ans;
}
// Driver code.
int main()
{
binomial();
double sinTheta = 0.5;
ll n = 10;
cout << findCosNTheta(sinTheta, n) << endl;
return 0;
}
Java
// Java Program to find the value of sin(n?)
public class GFG {
private static final int MAX = 16;
static long nCr[][] = new long [MAX][MAX];
// This function use to calculate the
// binomial coefficient upto 15
static void binomial()
{
// use simple DP to find coefficient
for (int i = 0; i < MAX; i++) {
for (int j = 0; j <= i; j++) {
if (j == 0 || j == i)
nCr[i][j] = 1;
else
nCr[i][j] = nCr[i - 1][j] + nCr[i - 1][j - 1];
}
}
}
// Function to find the value of
static double findCosNTheta(double sinTheta, int n)
{
// find cosTheta from sinTheta
double cosTheta = Math.sqrt(1 - sinTheta * sinTheta);
// store required answer
double ans = 0;
// use to toggle sign in sequence.
long toggle = 1;
for (int i = 1; i <= n; i += 2) {
ans = ans + nCr[n][i] * Math.pow(cosTheta, n - i)
* Math.pow(sinTheta, i) * toggle;
toggle = toggle * -1;
}
return ans;
}
// Driver code
public static void main (String args[]){
binomial();
double sinTheta = 0.5;
int n = 10;
System.out.println(findCosNTheta(sinTheta, n));
}
// This code is contributed by ANKITRAI1
}
Python3
# Python3 program to find the # value of sin(n-theta) import math MAX=16 nCr=[[0 for i in range(MAX)] for i in range(MAX)] # Function to calculate the binomial # coefficient upto 15 def binomial(): # use simple DP to find coefficient for i in range(MAX): for j in range(0,i+1): if j == 0 or j == i: nCr[i][j] = 1 else: nCr[i][j] = nCr[i - 1][j] + nCr[i - 1][j - 1] # Function to find the value of cos(n-theta) def findCosNTheta(sinTheta,n): # find sinTheta from sinTheta cosTheta = math.sqrt(1 - sinTheta * sinTheta) # to store required answer ans = 0 # use to toggle sign in sequence. toggle = 1 for i in range(1,n+1,2): ans = (ans + nCr[n][i]*(cosTheta**(n - i)) *(sinTheta**i) * toggle) toggle = toggle * -1 return ans # Driver code if __name__=='__main__': binomial() sinTheta = 0.5 n = 10 print(findCosNTheta(sinTheta, n)) # this code is contributed by sahilshelangia
C#
// C# Program to find the value of sin(n?)
using System;
class GFG
{
private static int MAX = 16;
static long[,] nCr = new long [MAX, MAX];
// This function use to calculate the
// binomial coefficient upto 15
static void binomial()
{
// use simple DP to find coefficient
for (int i = 0; i < MAX; i++)
{
for (int j = 0; j <= i; j++)
{
if (j == 0 || j == i)
nCr[i, j] = 1;
else
nCr[i, j] = nCr[i - 1, j] +
nCr[i - 1, j - 1];
}
}
}
// Function to find the value of cos(n-theta)
static double findCosNTheta(double sinTheta, int n)
{
// find cosTheta from sinTheta
double cosTheta = Math.Sqrt(1 - sinTheta *
sinTheta);
// store required answer
double ans = 0;
// use to toggle sign in sequence.
long toggle = 1;
for (int i = 1; i <= n; i += 2)
{
ans = ans + nCr[n, i] * Math.Pow(cosTheta, n - i) *
Math.Pow(sinTheta, i) * toggle;
toggle = toggle * -1;
}
return ans;
}
// Driver code
public static void Main ()
{
binomial();
double sinTheta = 0.5;
int n = 10;
Console.Write(findCosNTheta(sinTheta, n));
}
}
// This code is contributed by ChitraNayal
PHP
<?php
// PHP Program to find the value of sin(n?)
$MAX=16;
$nCr=array_fill(0,$MAX,array_fill(0,$MAX,0));
// This function use to calculate the
// binomial coefficient upto 15
function binomial()
{
global $MAX,$nCr;
// use simple DP to find coefficient
for ($i = 0; $i < $MAX; $i++) {
for ($j = 0; $j <= $i; $j++) {
if ($j == 0 || $j == $i)
$nCr[$i][$j] = 1;
else
$nCr[$i][$j] = $nCr[$i - 1][$j] + $nCr[$i - 1][$j - 1];
}
}
}
// Function to find the value of
function findCosNTheta($sinTheta,$n)
{
global $MAX,$nCr;
// find cosTheta from sinTheta
$cosTheta = sqrt(1 - $sinTheta * $sinTheta);
// store required answer
$ans = 0;
// use to toggle sign in sequence.
$toggle = 1;
for ($i = 1; $i <= $n; $i += 2) {
$ans = $ans + $nCr[$n][$i] * pow($cosTheta, $n - $i)
* pow($sinTheta, $i) * $toggle;
$toggle = $toggle * -1;
}
return $ans;
}
// Driver code.
binomial();
$sinTheta = 0.5;
$n = 10;
echo findCosNTheta($sinTheta, $n);
// this code is contributed by mits
?>
Javascript
<script>
// Javascript Program to find the value of sin(n?)
MAX = 16
var nCr = Array.from(Array(MAX), () => new Array(MAX));
// This function use to calculate the
// binomial coefficient upto 15
function binomial()
{
// use simple DP to find coefficient
for (var i = 0; i < MAX; i++) {
for (var j = 0; j <= i; j++) {
if (j == 0 || j == i)
nCr[i][j] = 1;
else
nCr[i][j] = nCr[i - 1][j] +
nCr[i - 1][j - 1];
}
}
}
// Function to find the value of
function findCosNTheta(sinTheta, n)
{
// find cosTheta from sinTheta
var cosTheta = Math.sqrt(1 - sinTheta * sinTheta);
// store required answer
var ans = 0;
// use to toggle sign in sequence.
var toggle = 1;
for (var i = 1; i <= n; i += 2) {
ans = ans + nCr[n][i] * Math.pow(cosTheta, n - i)
* Math.pow(sinTheta, i) * toggle;
toggle = toggle * -1;
}
return ans.toFixed(6);
}
// Driver code.
binomial();
var sinTheta = 0.5;
var n = 10;
document.write( findCosNTheta(sinTheta, n));
</script>
Producción:
-0.866025
Publicación traducida automáticamente
Artículo escrito por sahilshelangia y traducido por Barcelona Geeks. The original can be accessed here. Licence: CCBY-SA