Dado un círculo que tiene una cuerda en su interior. Se dan la longitud de la cuerda y el radio del círculo. La tarea es encontrar la distancia más corta desde la cuerda hasta el centro.
Ejemplos:
Input: r = 4, d = 3 Output: 3.7081 Input: r = 9.8, d = 7.3 Output: 9.09492
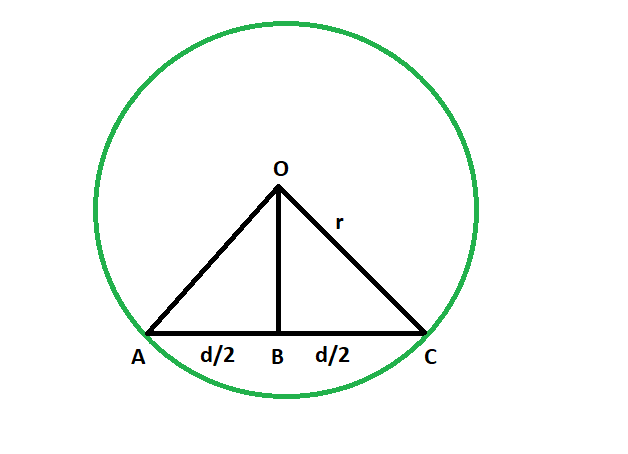
Enfoque :
- Sabemos que el segmento de línea que se deja caer desde el centro de la cuerda biseca la cuerda. La línea es la bisectriz perpendicular de la cuerda, y sabemos que la distancia perpendicular es la distancia más corta, por lo que nuestra tarea es encontrar la longitud de esta bisectriz perpendicular.
- sea radio del circulo = r
- longitud de la cuerda = d
- entonces, en el triángulo OBC ,
del teorema de Pitágoras ,
OB^2 + (d/2)^2 = r^2
entonces, OB = √(r^2 – d^2/4) - Asi que,
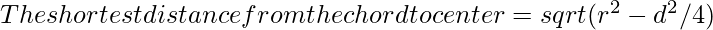
A continuación se muestra la implementación del enfoque anterior:
C++
// C++ program to find
// the shortest distance from
// chord to the center of circle
#include <bits/stdc++.h>
using namespace std;
// Function to find the shortest distance
void shortdis(double r, double d)
{
cout << "The shortest distance "
<< "from the chord to center "
<< sqrt((r * r) - ((d * d) / 4))
<< endl;
}
// Driver code
int main()
{
double r = 4, d = 3;
shortdis(r, d);
return 0;
}
Java
// Java program to find
// the shortest distance from
// chord to the center of circle
class GFG
{
// Function to find the shortest distance
static void shortdis(double r, double d)
{
System.out.println("The shortest distance "
+ "from the chord to center "
+ (Math.sqrt((r * r) - ((d * d) / 4))));
}
// Driver code
public static void main(String[] args)
{
double r = 4, d = 3;
shortdis(r, d);
}
}
/* This code contributed by PrinciRaj1992 */
Python3
# Python program to find
# the shortest distance from
# chord to the center of circle
# Function to find the shortest distance
def shortdis(r, d):
print("The shortest distance ",end="");
print("from the chord to center ",end="");
print(((r * r) - ((d * d) / 4))**(1/2));
# Driver code
r = 4;
d = 3;
shortdis(r, d);
# This code has been contributed by 29AjayKumar
C#
// C# program to find
// the shortest distance from
// chord to the center of circle
using System;
class GFG
{
// Function to find the shortest distance
static void shortdis(double r, double d)
{
Console.WriteLine("The shortest distance "
+ "from the chord to center "
+ (Math.Sqrt((r * r) - ((d * d) / 4))));
}
// Driver code
public static void Main()
{
double r = 4, d = 3;
shortdis(r, d);
}
}
// This code is contributed by AnkitRai01
PHP
<?php
// PHP program to find
// the shortest distance from
// chord to the center of circle
// Function to find the shortest distance
function shortdis($r, $d)
{
echo "The shortest distance ";
echo "from the chord to center ";
echo sqrt(($r * $r) - (($d * $d) / 4));
}
// Driver code
$r = 4;
$d = 3;
shortdis($r, $d);
// This code is contributed by Naman_Garg.
?>
Javascript
<script>
// JavaScript program to find
// the shortest distance from
// chord to the center of circle
// Function to find the shortest distance
function shortdis(r, d)
{
document.write("The shortest distance "
+ "from the chord to center "
+ Math.sqrt((r * r) - ((d * d) / 4))
+ "<br>");
}
// Driver code
let r = 4, d = 3;
shortdis(r, d);
// This code is contributed by Surbhi Tyagi.
</script>
Producción:
The shortest distance from the chord to centre 3.7081
Complejidad de tiempo: O(1)
Espacio Auxiliar: O(1)
Publicación traducida automáticamente
Artículo escrito por IshwarGupta y traducido por Barcelona Geeks. The original can be accessed here. Licence: CCBY-SA