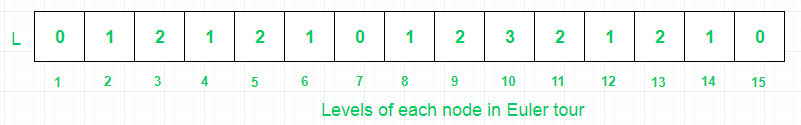
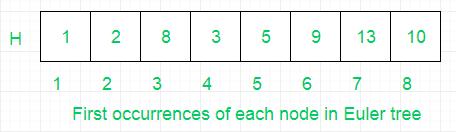
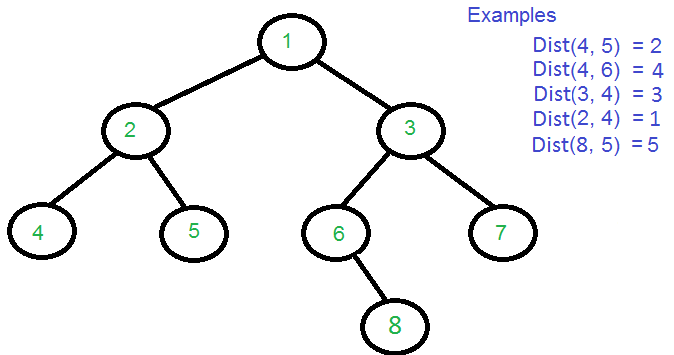
Dado un árbol binario, la tarea es encontrar la distancia entre dos claves en un árbol binario, no se dan punteros principales. La distancia entre dos Nodes es el número mínimo de aristas que se deben atravesar para llegar a un Node desde otro.
Este problema ya se discutió en una publicación anterior, pero utiliza tres recorridos del árbol binario, uno para encontrar el ancestro común más bajo (LCA) de dos Nodes (let A y B) y luego dos recorridos para encontrar la distancia entre LCA y A y LCA y B que tiene una complejidad temporal O(n). En esta publicación, se discutirá un método que requiere el tiempo O(log(n)) para encontrar LCA de dos Nodes.
La distancia entre dos Nodes se puede obtener en términos del ancestro común más bajo. La siguiente es la fórmula.
Dist(n1, n2) = Dist(root, n1) + Dist(root, n2) - 2*Dist(root, lca) 'n1' and 'n2' are the two given keys 'root' is root of given Binary Tree. 'lca' is lowest common ancestor of n1 and n2 Dist(n1, n2) is the distance between n1 and n2.
La fórmula anterior también se puede escribir como:
Dist(n1, n2) = Level[n1] + Level[n2] - 2*Level[lca]
Este problema se puede dividir en:
- Encontrar niveles de cada Node
- Encontrar el recorrido de Euler del árbol binario
- Árbol de segmentos de construcción para LCA,
Estos pasos se explican a continuación:
- Encuentre los niveles de cada Node aplicando un recorrido de orden de nivel .
- Encuentre el LCA de dos Nodes en un árbol binario en O (logn) almacenando el recorrido de Euler del árbol binario en una array y calculando otras dos arrays con la ayuda de los niveles de cada Node y el recorrido de Euler.
Estos pasos se muestran a continuación:
(I) Primero, encuentre el Tour de Euler del árbol binario.

Tour de Euler del árbol binario en el ejemplo
- (II) Luego, almacene los niveles de cada Node en la array de Euler en una array diferente.
- (III) Luego, almacene las primeras ocurrencias de todos los Nodes del árbol binario en la array de Euler. H almacena los índices de los Nodes de la array de Euler, por lo que el rango de consulta para encontrar el mínimo se puede minimizar y optimizar aún más el tiempo de consulta.
- Luego construya un árbol de segmentos en la array L y tome los valores bajos y altos de la array H que nos darán las primeras apariciones de, digamos, dos Nodes (A y B). Luego, consultamos el árbol de segmentos para encontrar el valor mínimo, digamos X en el rango ( H[A] a H[B] ). Luego usamos el índice del valor X como índice para la array de Euler para obtener LCA , es decir, Euler [índice (X)].
Sea A = 8 y B = 5.
(I) H[8] = 1 y H[5] =2
(II) Consultando en el árbol de segmentos, obtenemos el valor mínimo en la array L entre 1 y 2 como X=0, index=7
(III) Entonces, LCA= Euler[7], es decir, LCA = 1.- Finalmente, aplicamos la fórmula de distancia discutida anteriormente para obtener la distancia entre dos Nodes.
C++
// C++ program to find distance between
// two nodes for multiple queries
#include <bits/stdc++.h>
#define MAX 100001
using namespace std;
/* A tree node structure */
struct Node {
int data;
struct Node* left;
struct Node* right;
};
/* Utility function to create a new Binary Tree node */
struct Node* newNode(int data)
{
struct Node* temp = new struct Node;
temp->data = data;
temp->left = temp->right = NULL;
return temp;
}
// Array to store level of each node
int level[MAX];
// Utility Function to store level of all nodes
void FindLevels(struct Node* root)
{
if (!root)
return;
// queue to hold tree node with level
queue<pair<struct Node*, int> > q;
// let root node be at level 0
q.push({ root, 0 });
pair<struct Node*, int> p;
// Do level Order Traversal of tree
while (!q.empty()) {
p = q.front();
q.pop();
// Node p.first is on level p.second
level[p.first->data] = p.second;
// If left child exits, put it in queue
// with current_level +1
if (p.first->left)
q.push({ p.first->left, p.second + 1 });
// If right child exists, put it in queue
// with current_level +1
if (p.first->right)
q.push({ p.first->right, p.second + 1 });
}
}
// Stores Euler Tour
int Euler[MAX];
// index in Euler array
int idx = 0;
// Find Euler Tour
void eulerTree(struct Node* root)
{
// store current node's data
Euler[++idx] = root->data;
// If left node exists
if (root->left) {
// traverse left subtree
eulerTree(root->left);
// store parent node's data
Euler[++idx] = root->data;
}
// If right node exists
if (root->right) {
// traverse right subtree
eulerTree(root->right);
// store parent node's data
Euler[++idx] = root->data;
}
}
// checks for visited nodes
int vis[MAX];
// Stores level of Euler Tour
int L[MAX];
// Stores indices of first occurrence
// of nodes in Euler tour
int H[MAX];
// Preprocessing Euler Tour for finding LCA
void preprocessEuler(int size)
{
for (int i = 1; i <= size; i++) {
L[i] = level[Euler[i]];
// If node is not visited before
if (vis[Euler[i]] == 0) {
// Add to first occurrence
H[Euler[i]] = i;
// Mark it visited
vis[Euler[i]] = 1;
}
}
}
// Stores values and positions
pair<int, int> seg[4 * MAX];
// Utility function to find minimum of
// pair type values
pair<int, int> min(pair<int, int> a,
pair<int, int> b)
{
if (a.first <= b.first)
return a;
else
return b;
}
// Utility function to build segment tree
pair<int, int> buildSegTree(int low, int high, int pos)
{
if (low == high) {
seg[pos].first = L[low];
seg[pos].second = low;
return seg[pos];
}
int mid = low + (high - low) / 2;
buildSegTree(low, mid, 2 * pos);
buildSegTree(mid + 1, high, 2 * pos + 1);
seg[pos] = min(seg[2 * pos], seg[2 * pos + 1]);
}
// Utility function to find LCA
pair<int, int> LCA(int qlow, int qhigh, int low,
int high, int pos)
{
if (qlow <= low && qhigh >= high)
return seg[pos];
if (qlow > high || qhigh < low)
return { INT_MAX, 0 };
int mid = low + (high - low) / 2;
return min(LCA(qlow, qhigh, low, mid, 2 * pos),
LCA(qlow, qhigh, mid + 1, high, 2 * pos + 1));
}
// Function to return distance between
// two nodes n1 and n2
int findDistance(int n1, int n2, int size)
{
// Maintain original Values
int prevn1 = n1, prevn2 = n2;
// Get First Occurrence of n1
n1 = H[n1];
// Get First Occurrence of n2
n2 = H[n2];
// Swap if low > high
if (n2 < n1)
swap(n1, n2);
// Get position of minimum value
int lca = LCA(n1, n2, 1, size, 1).second;
// Extract value out of Euler tour
lca = Euler[lca];
// return calculated distance
return level[prevn1] + level[prevn2] - 2 * level[lca];
}
void preProcessing(Node* root, int N)
{
// Build Tree
eulerTree(root);
// Store Levels
FindLevels(root);
// Find L and H array
preprocessEuler(2 * N - 1);
// Build segment Tree
buildSegTree(1, 2 * N - 1, 1);
}
/* Driver function to test above functions */
int main()
{
int N = 8; // Number of nodes
/* Constructing tree given in the above figure */
Node* root = newNode(1);
root->left = newNode(2);
root->right = newNode(3);
root->left->left = newNode(4);
root->left->right = newNode(5);
root->right->left = newNode(6);
root->right->right = newNode(7);
root->right->left->right = newNode(8);
// Function to do all preprocessing
preProcessing(root, N);
cout << "Dist(4, 5) = " <<
findDistance(4, 5, 2 * N - 1) << "\n";
cout << "Dist(4, 6) = " <<
findDistance(4, 6, 2 * N - 1) << "\n";
cout << "Dist(3, 4) = " <<
findDistance(3, 4, 2 * N - 1) << "\n";
cout << "Dist(2, 4) = " <<
findDistance(2, 4, 2 * N - 1) << "\n";
cout << "Dist(8, 5) = " <<
findDistance(8, 5, 2 * N - 1) << "\n";
return 0;
}
Java
// Java program to find distance between
// two nodes for multiple queries
import java.io.*;
import java.util.*;
class GFG
{
static int MAX = 100001;
/* A tree node structure */
static class Node
{
int data;
Node left, right;
Node(int data)
{
this.data = data;
this.left = this.right = null;
}
}
static class Pair<T, V>
{
T first;
V second;
Pair() {
}
Pair(T first, V second)
{
this.first = first;
this.second = second;
}
}
// Array to store level of each node
static int[] level = new int[MAX];
// Utility Function to store level of all nodes
static void findLevels(Node root)
{
if (root == null)
return;
// queue to hold tree node with level
Queue<Pair<Node, Integer>> q = new LinkedList<>();
// let root node be at level 0
q.add(new Pair<Node, Integer>(root, 0));
Pair<Node, Integer> p = new Pair<Node, Integer>();
// Do level Order Traversal of tree
while (!q.isEmpty())
{
p = q.poll();
// Node p.first is on level p.second
level[p.first.data] = p.second;
// If left child exits, put it in queue
// with current_level +1
if (p.first.left != null)
q.add(new Pair<Node,
Integer>(p.first.left,
p.second + 1));
// If right child exists, put it in queue
// with current_level +1
if (p.first.right != null)
q.add(new Pair<Node,
Integer>(p.first.right,
p.second + 1));
}
}
// Stores Euler Tour
static int[] Euler = new int[MAX];
// index in Euler array
static int idx = 0;
// Find Euler Tour
static void eulerTree(Node root)
{
// store current node's data
Euler[++idx] = root.data;
// If left node exists
if (root.left != null)
{
// traverse left subtree
eulerTree(root.left);
// store parent node's data
Euler[++idx] = root.data;
}
// If right node exists
if (root.right != null)
{
// traverse right subtree
eulerTree(root.right);
// store parent node's data
Euler[++idx] = root.data;
}
}
// checks for visited nodes
static int[] vis = new int[MAX];
// Stores level of Euler Tour
static int[] L = new int[MAX];
// Stores indices of first occurrence
// of nodes in Euler tour
static int[] H = new int[MAX];
// Preprocessing Euler Tour for finding LCA
static void preprocessEuler(int size)
{
for (int i = 1; i <= size; i++)
{
L[i] = level[Euler[i]];
// If node is not visited before
if (vis[Euler[i]] == 0)
{
// Add to first occurrence
H[Euler[i]] = i;
// Mark it visited
vis[Euler[i]] = 1;
}
}
}
// Stores values and positions
@SuppressWarnings("unchecked")
static Pair<Integer, Integer>[] seg =
(Pair<Integer, Integer>[]) new Pair[4 * MAX];
// Utility function to find minimum of
// pair type values
static Pair<Integer, Integer>
min(Pair<Integer, Integer> a,
Pair<Integer, Integer> b)
{
if (a.first <= b.first)
return a;
return b;
}
// Utility function to build segment tree
static Pair<Integer, Integer> buildSegTree(int low,
int high, int pos)
{
if (low == high)
{
seg[pos].first = L[low];
seg[pos].second = low;
return seg[pos];
}
int mid = low + (high - low) / 2;
buildSegTree(low, mid, 2 * pos);
buildSegTree(mid + 1, high, 2 * pos + 1);
seg[pos] = min(seg[2 * pos], seg[2 * pos + 1]);
return seg[pos];
}
// Utility function to find LCA
static Pair<Integer, Integer> LCA(int qlow, int qhigh,
int low, int high, int pos)
{
if (qlow <= low && qhigh >= high)
return seg[pos];
if (qlow > high || qhigh < low)
return new Pair<Integer, Integer>
(Integer.MAX_VALUE, 0);
int mid = low + (high - low) / 2;
return min(LCA(qlow, qhigh, low, mid, 2 * pos),
LCA(qlow, qhigh, mid + 1, high, 2 * pos + 1));
}
// Function to return distance between
// two nodes n1 and n2
static int findDistance(int n1, int n2, int size)
{
// Maintain original Values
int prevn1 = n1, prevn2 = n2;
// Get First Occurrence of n1
n1 = H[n1];
// Get First Occurrence of n2
n2 = H[n2];
// Swap if low > high
if (n2 < n1)
{
int temp = n1;
n1 = n2;
n2 = temp;
}
// Get position of minimum value
int lca = LCA(n1, n2, 1, size, 1).second;
// Extract value out of Euler tour
lca = Euler[lca];
// return calculated distance
return level[prevn1] + level[prevn2] -
2 * level[lca];
}
static void preProcessing(Node root, int N)
{
for (int i = 0; i < 4 * MAX; i++)
{
seg[i] = new Pair<>();
}
// Build Tree
eulerTree(root);
// Store Levels
findLevels(root);
// Find L and H array
preprocessEuler(2 * N - 1);
// Build segment Tree
buildSegTree(1, 2 * N - 1, 1);
}
// Driver Code
public static void main(String[] args)
{
// Number of nodes
int N = 8;
/* Constructing tree given in the above figure */
Node root = new Node(1);
root.left = new Node(2);
root.right = new Node(3);
root.left.left = new Node(4);
root.left.right = new Node(5);
root.right.left = new Node(6);
root.right.right = new Node(7);
root.right.left.right = new Node(8);
// Function to do all preprocessing
preProcessing(root, N);
System.out.println("Dist(4, 5) = " +
findDistance(4, 5, 2 * N - 1));
System.out.println("Dist(4, 6) = " +
findDistance(4, 6, 2 * N - 1));
System.out.println("Dist(3, 4) = " +
findDistance(3, 4, 2 * N - 1));
System.out.println("Dist(2, 4) = " +
findDistance(2, 4, 2 * N - 1));
System.out.println("Dist(8, 5) = " +
findDistance(8, 5, 2 * N - 1));
}
}
// This code is contributed by
// sanjeev2552
Python3
# Python3 program to find distance between
# two nodes for multiple queries
from collections import deque
from sys import maxsize as INT_MAX
MAX = 100001
# A tree node structure
class Node:
def __init__(self, data):
self.data = data
self.left = None
self.right = None
# Array to store level of each node
level = [0] * MAX
# Utility Function to store level of all nodes
def findLevels(root: Node):
global level
if root is None:
return
# queue to hold tree node with level
q = deque()
# let root node be at level 0
q.append((root, 0))
# Do level Order Traversal of tree
while q:
p = q[0]
q.popleft()
# Node p.first is on level p.second
level[p[0].data] = p[1]
# If left child exits, put it in queue
# with current_level +1
if p[0].left:
q.append((p[0].left, p[1] + 1))
# If right child exists, put it in queue
# with current_level +1
if p[0].right:
q.append((p[0].right, p[1] + 1))
# Stores Euler Tour
Euler = [0] * MAX
# index in Euler array
idx = 0
# Find Euler Tour
def eulerTree(root: Node):
global Euler, idx
idx += 1
# store current node's data
Euler[idx] = root.data
# If left node exists
if root.left:
# traverse left subtree
eulerTree(root.left)
idx += 1
# store parent node's data
Euler[idx] = root.data
# If right node exists
if root.right:
# traverse right subtree
eulerTree(root.right)
idx += 1
# store parent node's data
Euler[idx] = root.data
# checks for visited nodes
vis = [0] * MAX
# Stores level of Euler Tour
L = [0] * MAX
# Stores indices of the first occurrence
# of nodes in Euler tour
H = [0] * MAX
# Preprocessing Euler Tour for finding LCA
def preprocessEuler(size: int):
global L, H, vis
for i in range(1, size + 1):
L[i] = level[Euler[i]]
# If node is not visited before
if vis[Euler[i]] == 0:
# Add to first occurrence
H[Euler[i]] = i
# Mark it visited
vis[Euler[i]] = 1
# Stores values and positions
seg = [0] * (4 * MAX)
for i in range(4 * MAX):
seg[i] = [0, 0]
# Utility function to find minimum of
# pair type values
def minPair(a: list, b: list) -> list:
if a[0] <= b[0]:
return a
else:
return b
# Utility function to build segment tree
def buildSegTree(low: int, high: int,
pos: int) -> list:
if low == high:
seg[pos][0] = L[low]
seg[pos][1] = low
return seg[pos]
mid = low + (high - low) // 2
buildSegTree(low, mid, 2 * pos)
buildSegTree(mid + 1, high, 2 * pos + 1)
seg[pos] = min(seg[2 * pos], seg[2 * pos + 1])
# Utility function to find LCA
def LCA(qlow: int, qhigh: int, low: int,
high: int, pos: int) -> list:
if qlow <= low and qhigh >= high:
return seg[pos]
if qlow > high or qhigh < low:
return [INT_MAX, 0]
mid = low + (high - low) // 2
return minPair(LCA(qlow, qhigh, low, mid, 2 * pos),
LCA(qlow, qhigh, mid + 1, high, 2 * pos + 1))
# Function to return distance between
# two nodes n1 and n2
def findDistance(n1: int, n2: int, size: int) -> int:
# Maintain original Values
prevn1 = n1
prevn2 = n2
# Get First Occurrence of n1
n1 = H[n1]
# Get First Occurrence of n2
n2 = H[n2]
# Swap if low>high
if n2 < n1:
n1, n2 = n2, n1
# Get position of minimum value
lca = LCA(n1, n2, 1, size, 1)[1]
# Extract value out of Euler tour
lca = Euler[lca]
# return calculated distance
return level[prevn1] + level[prevn2] -
2 * level[lca]
def preProcessing(root: Node, N: int):
# Build Tree
eulerTree(root)
# Store Levels
findLevels(root)
# Find L and H array
preprocessEuler(2 * N - 1)
# Build sparse table
buildSegTree(1, 2 * N - 1, 1)
# Driver Code
if __name__ == "__main__":
# Number of nodes
N = 8
# Constructing tree given in the above figure
root = Node(1)
root.left = Node(2)
root.right = Node(3)
root.left.left = Node(4)
root.left.right = Node(5)
root.right.left = Node(6)
root.right.right = Node(7)
root.right.left.right = Node(8)
# Function to do all preprocessing
preProcessing(root, N)
print("Dist(4, 5) =",
findDistance(4, 5, 2 * N - 1))
print("Dist(4, 6) =",
findDistance(4, 6, 2 * N - 1))
print("Dist(3, 4) =",
findDistance(3, 4, 2 * N - 1))
print("Dist(2, 4) =",
findDistance(2, 4, 2 * N - 1))
print("Dist(8, 5) =",
findDistance(8, 5, 2 * N - 1))
# This code is contributed by
# sanjeev2552
C#
// C# program to find distance between
// two nodes for multiple queries
using System;
using System.Collections.Generic;
class GFG
{
static int MAX = 100001;
/* A tree node structure */
public class Node
{
public int data;
public Node left, right;
public Node(int data)
{
this.data = data;
this.left = this.right = null;
}
}
class Pair<T, V>
{
public T first;
public V second;
public Pair() {
}
public Pair(T first, V second)
{
this.first = first;
this.second = second;
}
}
// Array to store level of each node
static int[] level = new int[MAX];
// Utility Function to store level of all nodes
static void findLevels(Node root)
{
if (root == null)
return;
// queue to hold tree node with level
List<Pair<Node, int>> q =
new List<Pair<Node, int>>();
// let root node be at level 0
q.Add(new Pair<Node, int>(root, 0));
Pair<Node, int> p = new Pair<Node, int>();
// Do level Order Traversal of tree
while (q.Count != 0)
{
p = q[0];
q.RemoveAt(0);
// Node p.first is on level p.second
level[p.first.data] = p.second;
// If left child exits, put it in queue
// with current_level +1
if (p.first.left != null)
q.Add(new Pair<Node, int>
(p.first.left, p.second + 1));
// If right child exists, put it in queue
// with current_level +1
if (p.first.right != null)
q.Add(new Pair<Node, int>
(p.first.right, p.second + 1));
}
}
// Stores Euler Tour
static int[] Euler = new int[MAX];
// index in Euler array
static int idx = 0;
// Find Euler Tour
static void eulerTree(Node root)
{
// store current node's data
Euler[++idx] = root.data;
// If left node exists
if (root.left != null)
{
// traverse left subtree
eulerTree(root.left);
// store parent node's data
Euler[++idx] = root.data;
}
// If right node exists
if (root.right != null)
{
// traverse right subtree
eulerTree(root.right);
// store parent node's data
Euler[++idx] = root.data;
}
}
// checks for visited nodes
static int[] vis = new int[MAX];
// Stores level of Euler Tour
static int[] L = new int[MAX];
// Stores indices of first occurrence
// of nodes in Euler tour
static int[] H = new int[MAX];
// Preprocessing Euler Tour for finding LCA
static void preprocessEuler(int size)
{
for (int i = 1; i <= size; i++)
{
L[i] = level[Euler[i]];
// If node is not visited before
if (vis[Euler[i]] == 0)
{
// Add to first occurrence
H[Euler[i]] = i;
// Mark it visited
vis[Euler[i]] = 1;
}
}
}
// Stores values and positions
static Pair<int, int>[] seg = new
Pair<int, int>[4 * MAX];
// Utility function to find minimum of
// pair type values
static Pair<int, int> min(Pair<int, int> a,
Pair<int, int> b)
{
if (a.first <= b.first)
return a;
return b;
}
// Utility function to build segment tree
static Pair<int, int> buildSegTree(int low,
int high, int pos)
{
if (low == high)
{
seg[pos].first = L[low];
seg[pos].second = low;
return seg[pos];
}
int mid = low + (high - low) / 2;
buildSegTree(low, mid, 2 * pos);
buildSegTree(mid + 1, high, 2 * pos + 1);
seg[pos] = min(seg[2 * pos], seg[2 * pos + 1]);
return seg[pos];
}
// Utility function to find LCA
static Pair<int, int> LCA(int qlow, int qhigh,
int low, int high, int pos)
{
if (qlow <= low && qhigh >= high)
return seg[pos];
if (qlow > high || qhigh < low)
return new Pair<int, int>(int.MaxValue, 0);
int mid = low + (high - low) / 2;
return min(LCA(qlow, qhigh, low, mid, 2 * pos),
LCA(qlow, qhigh, mid + 1,
high, 2 * pos + 1));
}
// Function to return distance between
// two nodes n1 and n2
static int findDistance(int n1, int n2, int size)
{
// Maintain original Values
int prevn1 = n1, prevn2 = n2;
// Get First Occurrence of n1
n1 = H[n1];
// Get First Occurrence of n2
n2 = H[n2];
// Swap if low > high
if (n2 < n1)
{
int temp = n1;
n1 = n2;
n2 = temp;
}
// Get position of minimum value
int lca = LCA(n1, n2, 1, size, 1).second;
// Extract value out of Euler tour
lca = Euler[lca];
// return calculated distance
return level[prevn1] + level[prevn2] -
2 * level[lca];
}
static void preProcessing(Node root, int N)
{
for (int i = 0; i < 4 * MAX; i++)
{
seg[i] = new Pair<int,int>();
}
// Build Tree
eulerTree(root);
// Store Levels
findLevels(root);
// Find L and H array
preprocessEuler(2 * N - 1);
// Build segment Tree
buildSegTree(1, 2 * N - 1, 1);
}
// Driver Code
public static void Main(String[] args)
{
// Number of nodes
int N = 8;
/* Constructing tree given in the above figure */
Node root = new Node(1);
root.left = new Node(2);
root.right = new Node(3);
root.left.left = new Node(4);
root.left.right = new Node(5);
root.right.left = new Node(6);
root.right.right = new Node(7);
root.right.left.right = new Node(8);
// Function to do all preprocessing
preProcessing(root, N);
Console.WriteLine("Dist(4, 5) = " +
findDistance(4, 5, 2 * N - 1));
Console.WriteLine("Dist(4, 6) = " +
findDistance(4, 6, 2 * N - 1));
Console.WriteLine("Dist(3, 4) = " +
findDistance(3, 4, 2 * N - 1));
Console.WriteLine("Dist(2, 4) = " +
findDistance(2, 4, 2 * N - 1));
Console.WriteLine("Dist(8, 5) = " +
findDistance(8, 5, 2 * N - 1));
}
}
// This code is contributed by Rajput-Ji
Salida :
Dist(4, 5) = 2 Dist(4, 6) = 4 Dist(3, 4) = 3 Dist(2, 4) = 1 Dist(8, 5) = 5
Complejidad de tiempo: O(Log N)
Complejidad de espacio: O(N)
Consultas para encontrar la distancia entre dos Nodes de un árbol binario – método O(1)
Publicación traducida automáticamente
Artículo escrito por Abhishek rajput y traducido por Barcelona Geeks. The original can be accessed here. Licence: CCBY-SA