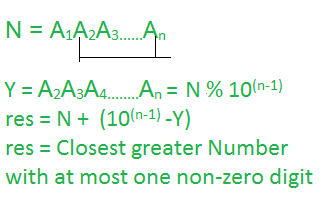
Dado un número entero N , la tarea es encontrar el número más cercano a N que sea mayor que N y que contenga como máximo un dígito distinto de cero .
Ejemplos:
Entrada : N = 540
Salida: 600
Explicación: Dado que el número 600 contiene solo un dígito distinto de cero, la salida requerida es 600.Entrada : N = 1000
Salida: 2000
Enfoque: El problema se puede resolver con base en las siguientes observaciones.
Siga los pasos a continuación para resolver el problema:
- Inicialice una variable, por ejemplo, ctr para almacenar el recuento de dígitos en N .
- Calcule el valor de la potencia (10, ctr – 1 )
- Imprima el valor de la fórmula mencionada anteriormente como la respuesta requerida.
A continuación se muestra la implementación del enfoque anterior:
C++
// C++ program to implement
// the above approach
#include <bits/stdc++.h>
using namespace std;
// Function to calculate
// X ^ n in log(n)
int power(int X, int n) {
// Stores the value
// of X^n
int res = 1;
while(n) {
// If N is odd
if(n & 1)
res = res * X;
X = X * X;
n = n >> 1;
}
return res;
}
// Function to find the
// closest number > N having
// at most 1 non-zero digit
int closestgtNum(int N) {
// Stores the count
// of digits in N
int n = log10(N) + 1;
// Stores the power
// of 10^(n-1)
int P = power(10, n - 1);
// Stores the
// last (n - 1) digits
int Y = N % P;
// Store the answer
int res = N + (P - Y);
return res;
}
// Driver Code
int main()
{
int N = 120;
cout<<closestgtNum(N);
}
Java
// Java program to implement
// the above approach
import java.io.*;
class GFG{
// Function to calculate
// X ^ n in log(n)
static int power(int X, int n)
{
// Stores the value
// of X^n
int res = 1;
while(n != 0)
{
// If N is odd
if ((n & 1) != 0)
res = res * X;
X = X * X;
n = n >> 1;
}
return res;
}
// Function to find the
// closest number > N having
// at most 1 non-zero digit
static int closestgtNum(int N)
{
// Stores the count
// of digits in N
int n = (int) Math.log10(N) + 1;
// Stores the power
// of 10^(n-1)
int P = power(10, n - 1);
// Stores the
// last (n - 1) digits
int Y = N % P;
// Store the answer
int res = N + (P - Y);
return res;
}
// Driver Code
public static void main (String[] args)
{
int N = 120;
// Function call
System.out.print(closestgtNum(N));
}
}
// This code is contributed by code_hunt
Python3
# Python3 program to implement # the above approach import math # Function to calculate # X ^ n in log(n) def power(X, n): # Stores the value # of X^n res = 1 while (n != 0): # If N is odd if (n & 1 != 0): res = res * X X = X * X n = n >> 1 return res # Function to find the # closest number > N having # at most 1 non-zero digit def closestgtNum(N): # Stores the count # of digits in N n = int(math.log10(N) + 1) # Stores the power # of 10^(n-1) P = power(10, n - 1) # Stores the # last (n - 1) digits Y = N % P # Store the answer res = N + (P - Y) return res # Driver Code N = 120 print(closestgtNum(N)) # This code is contributed by code_hunt
C#
// C# program to implement
// the above approach
using System;
class GFG{
// Function to calculate
// X ^ n in log(n)
static int power(int X, int n)
{
// Stores the value
// of X^n
int res = 1;
while(n != 0)
{
// If N is odd
if ((n & 1) != 0)
res = res * X;
X = X * X;
n = n >> 1;
}
return res;
}
// Function to find the
// closest number > N having
// at most 1 non-zero digit
static int closestgtNum(int N)
{
// Stores the count
// of digits in N
int n = (int) Math.Log10(N) + 1;
// Stores the power
// of 10^(n-1)
int P = power(10, n - 1);
// Stores the
// last (n - 1) digits
int Y = N % P;
// Store the answer
int res = N + (P - Y);
return res;
}
// Driver Code
public static void Main ()
{
int N = 120;
// Function call
Console.Write(closestgtNum(N));
}
}
// This code is contributed by code_hunt
Javascript
<script>
// JavaScript program to implement
// the above approach
// Function to calculate
// X ^ n in log(n)
function power(X, n)
{
// Stores the value
// of X^n
var res = 1;
while(n != 0)
{
// If N is odd
if ((n & 1) != 0)
res = res * X;
X = X * X;
n = n >> 1;
}
return res;
}
// Function to find the
// closest number > N having
// at most 1 non-zero digit
function closestgtNum(N)
{
// Stores the count
// of digits in N
var n = parseInt( Math.log10(N) + 1);
// Stores the power
// of 10^(n-1)
var P = power(10, n - 1);
// Stores the
// last (n - 1) digits
var Y = N % P;
// Store the answer
var res = N + (P - Y);
return res;
}
// Driver Code
var N = 120;
// Function call
document.write(closestgtNum(N));
</script>
200
Complejidad de tiempo: O(log 2 N)
Espacio auxiliar: O(log 10 N)
Enfoque eficiente: la idea es incrementar el valor del primer dígito del entero dado en 1 e inicializar la string resultante al primer dígito del entero dado. Finalmente, agregue (N – 1) 0 s al final de la string resultante y devuelva la string resultante.
- Inicialice una string, diga res para almacenar el número mayor más cercano con st más un dígito distinto de cero.
- Primero agregue el valor str[0] + 1 en la string resultante y luego agregue (N – 1) 0 s al final de la string resultante.
- Imprimir el valor de res
A continuación se muestra la implementación del enfoque anterior:
C++
// C++ program to implement
// the above approach
#include <bits/stdc++.h>
using namespace std;
// Function to get closest greater
// number with at most non zero digit
string closestgtNum(string str)
{
// Stores the closest greater number
// with at most one non-zero digit
string res = "";
// Stores length of str
int n = str.length();
if(str[0] < '9') {
res.push_back(str[0] + 1);
}
else{
// Append 10 to the end
// of resultant string
res.push_back('1');
res.push_back('0');
}
// Append n-1 times '0' to the end
// of resultant string
for(int i = 0; i < n - 1; i++)
{
res.push_back('0');
}
return res;
}
// Driver Code
int main()
{
string str = "120";
cout<<closestgtNum(str);
}
Java
// Java program to implement
// the above approach
import java.util.*;
class GFG{
// Function to get closest greater
// number with at most non zero digit
static String closestgtNum(String str)
{
// Stores the closest greater number
// with at most one non-zero digit
String res = "";
// Stores length of str
int n = str.length();
if (str.charAt(0) < '9')
{
res += (char)(str.charAt(0) + 1);
}
else
{
// Append 10 to the end
// of resultant String
res += (char)('1');
res += (char)('0');
}
// Append n-1 times '0' to the end
// of resultant String
for(int i = 0; i < n - 1; i++)
{
res += (char)('0');
}
return res;
}
// Driver Code
public static void main(String[] args)
{
String str = "120";
System.out.print(closestgtNum(str));
}
}
// This code is contributed by Amit Katiyar
Python3
# Python3 program to implement
# the above approach
# Function to get closest greater
# number with at most non zero digit
def closestgtNum(str):
# Stores the closest greater number
# with at most one non-zero digit
res = "";
# Stores length of str
n = len(str);
if (str[0] < '9'):
res += (chr)(ord(str[0]) + 1);
else:
# Append 10 to the end
# of resultant String
res += (chr)('1');
res += (chr)('0');
# Append n-1 times '0' to the end
# of resultant String
for i in range(n - 1):
res += ('0');
return res;
# Driver Code
if __name__ == '__main__':
str = "120";
print(closestgtNum(str));
# This code is contributed by Amit Katiyar
C#
// C# program to implement
// the above approach
using System;
class GFG{
// Function to get closest greater
// number with at most non zero digit
public static string closestgtNum(string str)
{
// Stores the closest greater number
// with at most one non-zero digit
string res = "";
// Stores length of str
int n = str.Length;
if (str[0] < '9')
{
res = res + (char)(str[0] + 1);
}
else
{
// Append 10 to the end
// of resultant string
res = res + '1';
res = res + '0';
}
// Append n-1 times '0' to the end
// of resultant string
for(int i = 0; i < n - 1; i++)
{
res = res + '0';
}
return res;
}
// Driver code
static void Main()
{
string str = "120";
Console.WriteLine(closestgtNum(str));
}
}
// This code is contributed by divyeshrabadiya07
Javascript
<script>
// Javascript program to implement
// the above approach
// Function to get closest greater
// number with at most non zero digit
function closestgtNum(str)
{
// Stores the closest greater number
// with at most one non-zero digit
var res = "";
// Stores length of str
var n = str.length;
if (str[0] < '9')
{
res = res + String.fromCharCode(str[0].charCodeAt(0) + 1);
}
else
{
// Append 10 to the end
// of resultant string
res = res + '1';
res = res + '0';
}
// Append n-1 times '0' to the end
// of resultant string
for(var i = 0; i < n - 1; i++)
{
res = res + '0';
}
return res;
}
// Driver code
var str = "120";
document.write(closestgtNum(str));
// This code is contributed by itsok.
</script>
200
Complejidad de tiempo: O(log 10 N)
Espacio auxiliar: O(log 10 N)
Publicación traducida automáticamente
Artículo escrito por aanchaltiwari y traducido por Barcelona Geeks. The original can be accessed here. Licence: CCBY-SA