Número máximo de caballos no atacantes que se pueden colocar en un tablero de ajedrez N*M

Posted on by Rudeus Greyrat

Dado un tablero de ajedrez N*M . La tarea es encontrar el número máximo de caballos que se pueden colocar en el tablero de ajedrez dado de modo que ningún caballo ataque a otro caballo.

Ejemplo

Entrada: N = 1, M = 4
Salida: 4
Coloque un caballo en cada celda del tablero de ajedrez.

Entrada: N = 4, M = 5
Salida: 10

Planteamiento: Como sabemos que un caballero puede atacar de dos formas. Estos son los lugares que puede atacar.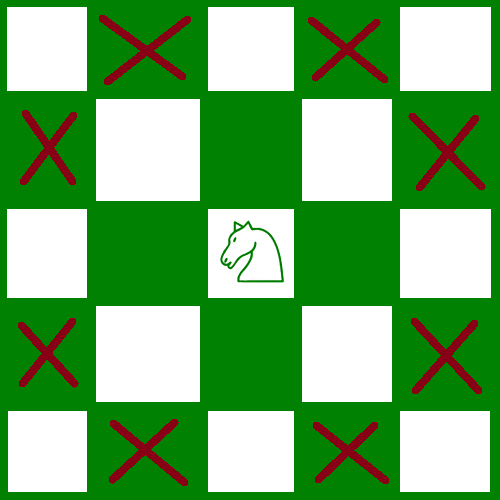

Aquí, en la imagen, el caballo está en color blanco y ataca solo al color negro. De este modo. llegamos a la conclusión de que un caballo puede atacar solo en un color diferente.
Podemos tomar la ayuda de este hecho y usarlo para nuestro propósito. Ahora que sabemos que los caballeros atacan en diferentes colores, podemos mantener a todos los caballeros en el mismo color, es decir, todos en blanco o todos en negro. Haciendo así el mayor número de caballos que se pueden colocar.
Para encontrar el número de blanco o negro, es simplemente la mitad del total de bloques a bordo.

Bloques totales = n * m
Bloques del mismo color = (n * m) / 2

Casos de esquina:

  • Si solo hay una sola fila o columna. Entonces todos los bloques pueden ser llenados por caballeros ya que un caballero no puede atacar en la misma fila o columna.
  • Si solo hay dos filas o columnas. Luego, cada dos columnas (o filas) se llenarán de caballos y cada dos columnas (o filas) consecutivas permanecerán vacías. Como se demuestra en la imagen.

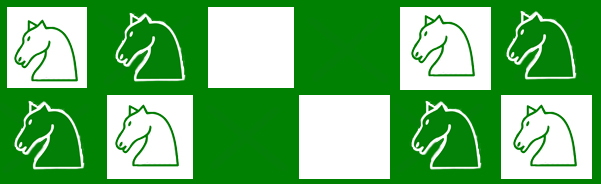
      • A continuación se muestra la implementación del enfoque anterior:

      C++

      // C++ implementation of the approach
      #include <bits/stdc++.h>
      using namespace std;
        
      // Function to return the maximum number of
      // knights that can be placed on the given
      // chessboard such that no two
      // knights attack each other
      int max_knight(int n, int m)
      {
        
          // Check for corner case #1
          // If row or column is 1
          if (m == 1 || n == 1) {
        
              // If yes, then simply print total blocks
              // which will be the max of row or column
              return max(m, n);
          }
        
          // Check for corner case #2
          // If row or column is 2
          else if (m == 2 || n == 2) {
        
              // If yes, then simply calculate
              // consecutive 2 rows or columns
              int c = 0;
              c = (max(m, n) / 4) * 4;
        
              if (max(m, n) % 4 == 1) {
                  c += 2;
              }
              else if (max(m, n) % 4 > 1) {
                  c += 4;
              }
              return c;
          }
        
          // For general case, just print the
          // half of total blocks
          else {
              return (((m * n) + 1) / 2);
          }
      }
        
      // Driver code
      int main()
      {
          int n = 4, m = 5;
        
          cout << max_knight(n, m);
        
          return 0;
      }

      Java

      // Java implementation of the approach 
      import java.io.*;
        
      class GFG 
      {
            
      // Function to return the maximum number of 
      // knights that can be placed on the given 
      // chessboard such that no two 
      // knights attack each other 
      static int max_knight(int n, int m) 
      { 
        
          // Check for corner case #1 
          // If row or column is 1 
          if (m == 1 || n == 1) 
          { 
        
              // If yes, then simply print total blocks 
              // which will be the max of row or column 
              return Math.max(m, n); 
          } 
        
          // Check for corner case #2 
          // If row or column is 2 
          else if (m == 2 || n == 2) 
          { 
        
              // If yes, then simply calculate 
              // consecutive 2 rows or columns 
              int c = 0; 
              c = (Math.max(m, n) / 4) * 4; 
        
              if (Math.max(m, n) % 4 == 1)
              { 
                  c += 2; 
              } 
              else if (Math.max(m, n) % 4 > 1) 
              { 
                  c += 4; 
              } 
              return c; 
          } 
        
          // For general case, just print the 
          // half of total blocks 
          else 
          { 
              return (((m * n) + 1) / 2); 
          } 
      } 
        
      // Driver code 
      public static void main (String[] args) 
      {
          int n = 4, m = 5; 
          System.out.println (max_knight(n, m)); 
      }
      }
        
      // This code is contributed by ajit 

      Python3

      # Python3 implementation of the approach 
        
      # Function to return the maximum number of 
      # knights that can be placed on the given 
      # chessboard such that no two 
      # knights attack each other 
      def max_knight(n, m) : 
        
          # Check for corner case #1 
          # If row or column is 1 
          if (m == 1 or n == 1) :
        
              # If yes, then simply print total blocks 
              # which will be the max of row or column 
              return max(m, n); 
        
          # Check for corner case #2 
          # If row or column is 2 
          elif (m == 2 or n == 2) :
        
              # If yes, then simply calculate 
              # consecutive 2 rows or columns 
              c = 0; 
              c = (max(m, n) // 4) * 4; 
        
              if (max(m, n) % 4 == 1) :
                  c += 2; 
                
              elif (max(m, n) % 4 > 1) :
                  c += 4; 
        
              return c; 
        
          # For general case, just print the 
          # half of total blocks 
          else :
              return (((m * n) + 1) // 2); 
        
      # Driver code 
      if __name__ == "__main__" : 
        
          n = 4; m = 5; 
        
          print(max_knight(n, m)); 
        
      # This code is contributed by AnkitRai01

      C#

      // C# implementation of the approach 
      using System;
        
      class GFG
      {
                
      // Function to return the maximum number of 
      // knights that can be placed on the given 
      // chessboard such that no two 
      // knights attack each other 
      static int max_knight(int n, int m) 
      { 
        
          // Check for corner case #1 
          // If row or column is 1 
          if (m == 1 || n == 1) 
          { 
        
              // If yes, then simply print total blocks 
              // which will be the max of row or column 
              return Math.Max(m, n); 
          } 
        
          // Check for corner case #2 
          // If row or column is 2 
          else if (m == 2 || n == 2) 
          { 
        
              // If yes, then simply calculate 
              // consecutive 2 rows or columns 
              int c = 0; 
              c = (Math.Max(m, n) / 4) * 4; 
        
              if (Math.Max(m, n) % 4 == 1)
              { 
                  c += 2; 
              } 
              else if (Math.Max(m, n) % 4 > 1) 
              { 
                  c += 4; 
              } 
              return c; 
          } 
        
          // For general case, just print the 
          // half of total blocks 
          else
          { 
              return (((m * n) + 1) / 2); 
          } 
      } 
        
      // Driver code 
      static public void Main()
      {
          int n = 4, m = 5; 
          Console.Write(max_knight(n, m)); 
      }
      }
        
      // This code is contributed by Tushil.
      Producción:

      10

Publicación traducida automáticamente

Artículo escrito por ShivamChauhan5 y traducido por Barcelona Geeks. The original can be accessed here. Licence: CCBY-SA

Deja una respuesta

Tu dirección de correo electrónico no será publicada. Los campos obligatorios están marcados con *