Dado un valor K y n, la tarea es encontrar la suma de la siguiente serie:
K n + ( K (n-1) * (K-1) 1 ) + ( K (n-2) * (K-1) 2 ) + ……. (K-1) norte
Ejemplos:
Input: n = 3, K = 3 Output: 65 Explanation: (3*3*3) + (3*3*2) + (3*2*2) + (2*2*2) = 27 + 18 + 12 + 8 = 65 Input: n = 4, k = 2 Output: 31 Explanation: (2*2*2*2) + (2*2*2*1)+ (2*2*1*1) + (2*1*1*1) + (1*1*1*1) = 16 + 8 + 4 + 2 + 1 = 31
- Enfoque simple: O(n 2 )
- Número total de términos en serie = n+1
- Calcula cada término por separado y súmalos:
- Segundo Enfoque: O(n)
Se observa que, dada la serie es Progresión Geométrica , con razón común = (K – 1)/K
Entonces, la expresión anterior se puede simplificar como:
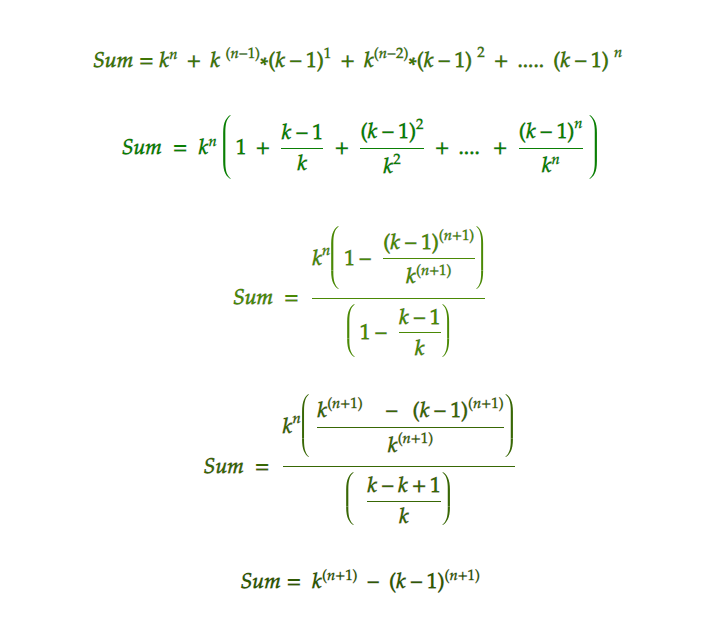
- A continuación se muestra la implementación del enfoque anterior:
C++
#include <bits/stdc++.h>
using namespace std;
// Function to return sum
int sum(int k, int n)
{
int sum
= pow(k, n + 1)
- pow(k - 1, n + 1);
return sum;
}
// Driver code
int main()
{
int n = 3;
int K = 3;
cout << sum(K, n);
}
Java
// Java implementation of above approach
class GFG
{
// Function to return sum
static int sum(int k, int n)
{
int sum = (int)(Math.pow(k, n + 1) -
Math.pow(k - 1, n + 1));
return sum;
}
// Driver code
public static void main(String args[])
{
int n = 3;
int K = 3;
System.out.print(sum(K, n));
}
}
// This code is contributed
// by Akanksha Rai
Python3
# Function to return sum def sum(k, n): sum = (pow(k, n + 1) - pow(k - 1, n + 1)); return sum; # Driver code n = 3; K = 3; print(sum(K, n)); # This code is contributed by mits
C#
// C# implementation of above approach
using System;
class GFG
{
// Function to return sum
static int sum(int k, int n)
{
int sum = (int)(Math.Pow(k, n + 1) -
Math.Pow(k - 1, n + 1));
return sum;
}
// Driver code
public static void Main()
{
int n = 3;
int K = 3;
Console.Write(sum(K, n));
}
}
// This code is contributed
// by Akanksha Rai
PHP
<?php
// Function to return sum
function sum($k, $n)
{
$sum = pow($k, $n + 1) -
pow($k - 1, $n + 1);
return $sum;
}
// Driver code
$n = 3;
$K = 3;
echo sum($K, $n);
// This code is contributed
// by Akanksha Rai
Javascript
<script>
// Javascript implementation of the approach
// Function to return sum
function sum(k,n)
{
let sum = 0;
for (let i = 0; i <= n; i++) {
let p = 1;
for (let j = 0; j < n - i; j++) {
p = p * k;
}
for (let j = 0; j < i; j++) {
p = p * (k - 1);
}
sum = sum + p;
}
return sum;
}
// Driver code
let n = 3;
let K = 3;
document.write(sum(K, n));
// This code is contributed by unknown2108
</script>
Producción:
65
Complejidad temporal: O( n )
Espacio Auxiliar: O(1)
- Tercer enfoque (eficiente): O(log n)
Nota: La complejidad del tiempo se puede reducir aún más a O(log(n)), calculando la potencia en complejidad log(n).
A continuación se muestra la implementación del enfoque anterior:
C++
#include <bits/stdc++.h>
using namespace std;
// Recursive C program to compute modular power
int exponent(int A, int B)
{
// Base cases
if (A == 0)
return 0;
if (B == 0)
return 1;
// If B is even
long y;
if (B % 2 == 0) {
y = exponent(A, B / 2);
y = (y * y);
}
// If B is odd
else {
y = A;
y = (y * exponent(A, B - 1));
}
return y;
}
// Function to return sum
int sum(int k, int n)
{
int sum = exponent(k, n + 1)
- exponent(k - 1, n + 1);
return sum;
}
// Driver code
int main()
{
int n = 3;
int K = 3;
cout << sum(K, n);
}
Java
import java.lang.Math;
class GFG
{
// Recursive C program to compute modular power
static int exponent(int A, int B)
{
// Base cases
if (A == 0)
return 0;
if (B == 0)
return 1;
// If B is even
int y;
if (B % 2 == 0)
{
y = exponent(A, B / 2);
y = (y * y);
}
// If B is odd
else
{
y = A;
y = (y * exponent(A, B - 1));
}
return y;
}
// Function to return sum
static int sum(int k, int n)
{
int sum = exponent(k, n + 1)
- exponent(k - 1, n + 1);
return sum;
}
// Driver code
public static void main(String[] args)
{
int n = 3;
int K = 3;
System.out.println(sum(K, n));
}
}
// This code is contributed by Code_Mech.
Python3
# Recursive python3 program to compute modular power def exponent(A, B): # Base cases if (A == 0): return 0; if (B == 0): return 1; # If B is even if (B % 2 == 0): y = exponent(A, B / 2); y = (y * y); # If B is odd else: y = A; y = (y * exponent(A, B - 1)); return y; # Function to return sum def sum(k, n): sum = exponent(k, n + 1) - exponent(k - 1, n + 1); return sum; # Driver code n = 3; K = 3; print(sum(K, n)); # This code has been contributed by 29AjayKumar
C#
// C# program of above approach
using System;
class GFG
{
// Recursive C program to compute modular power
static int exponent(int A, int B)
{
// Base cases
if (A == 0)
return 0;
if (B == 0)
return 1;
// If B is even
int y;
if (B % 2 == 0)
{
y = exponent(A, B / 2);
y = (y * y);
}
// If B is odd
else
{
y = A;
y = (y * exponent(A, B - 1));
}
return y;
}
// Function to return sum
static int sum(int k, int n)
{
int sum = exponent(k, n + 1)
- exponent(k - 1, n + 1);
return sum;
}
// Driver code
public static void Main()
{
int n = 3;
int K = 3;
Console.WriteLine(sum(K, n));
}
}
// This code is contributed by Code_Mech.
PHP
<?php
// Recursive C program to compute modular power
function exponent($A, $B)
{
// Base cases
if ($A == 0)
return 0;
if ($B == 0)
return 1;
// If B is even
if ($B % 2 == 0)
{
$y = exponent($A, $B / 2);
$y = ($y * $y);
}
// If B is odd
else
{
$y = $A;
$y = ($y * exponent($A, $B - 1));
}
return $y;
}
// Function to return sum
function sum($k, $n)
{
$sum = exponent($k, $n + 1) -
exponent($k - 1, $n + 1);
return $sum;
}
// Driver code
$n = 3;
$K = 3;
echo sum($K, $n);
// This code is contributed by Akanksha Rai
?>
Javascript
<script>
// Recursive Javascript program to
// compute modular power
function exponent(A, B)
{
// Base cases
if (A == 0)
return 0;
if (B == 0)
return 1;
// If B is even
let y;
if (B % 2 == 0)
{
y = exponent(A, parseInt(B / 2, 10));
y = (y * y);
}
// If B is odd
else
{
y = A;
y = (y * exponent(A, B - 1));
}
return y;
}
// Function to return sum
function sum(k, n)
{
let sum = exponent(k, n + 1) -
exponent(k - 1, n + 1);
return sum;
}
// Driver code
let n = 3;
let K = 3;
document.write(sum(K, n));
// This code is contributed by divyeshrabadiya07
</script>
Producción:
65
Complejidad de tiempo: O (logn)
Espacio auxiliar: O (logn)